| Date | May 2014 | Marks available | 16 | Reference code | 14M.3sp.hl.TZ0.2 |
| Level | HL only | Paper | Paper 3 Statistics and probability | Time zone | TZ0 |
| Command term | Determine, Estimate, Find, Hence, and State | Question number | 2 | Adapted from | N/A |
Question
The following table gives the average yield of olives per tree, in kg, and the rainfall, in cm, for nine separate regions of Greece. You may assume that these data are a random sample from a bivariate normal distribution, with correlation coefficient ρ.
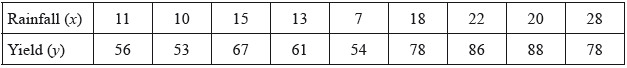
A scientist wishes to use these data to determine whether there is a positive correlation between rainfall and yield.
(a) State suitable hypotheses.
(b) Determine the product moment correlation coefficient for these data.
(c) Determine the associated p-value and comment on this value in the context of the question.
(d) Find the equation of the regression line of y on x.
(e) Hence, estimate the yield per tree in a tenth region where the rainfall was 19 cm.
(f) Determine the angle between the regression line of y on x and that of x on y . Give your answer to the nearest degree.
Markscheme
(a) H0:ρ=0 A1
H1:ρ>0 A1
[2 marks]
(b) 0.853 A2
Note: Accept any answer that rounds to 0.85.
[2 marks]
(c) p-value = 0.00173 (1-tailed) A1
Note: Accept any answer that rounds to 0.0017.
Accept any answer that rounds to 0.0035 obtained from 2-tailed test.
strong evidence to reject the hypothesis that there is no correlation between rainfall and yield or to accept the hypothesis that there is correlation between rainfall and yield R1
Note: Follow through the p-value for the conclusion.
[2 marks]
(d) y=1.78x+40.5 A1A1
Note: Accept numerical coefficients that round to 1.8 and 41.
[2 marks]
(e) y=1.77…(19)+14.5… M1
74.3 A1
Note: Accept any answer that rounds to 74 or 75.
[2 marks]
(f) the gradient of the regression line y on x is 1.78 or equivalent A1
the regression line of x on y is x=0.409y−12.2 (A1)
the gradient of the regression line x on y is 10.409 (=2.44) (M1)A1
calculate arctan(2.44)−arctan(1.78) (M1)
angle between regression lines is 7 degrees A1
Note: Accept any answer which rounds to ±7 degrees.
[6 marks]
Total [16 marks]

