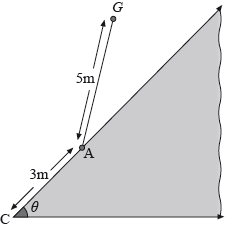
The diagram below shows a fenced triangular enclosure in the middle of a large grassy field. The points A and C are 3 m apart. A goat \(G\) is tied by a 5 m length of rope at point A on the outside edge of the enclosure.

Given that the corner of the enclosure at C forms an angle of \(\theta \) radians and the area of field that can be reached by the goat is \({\text{44 }}{{\text{m}}^{\text{2}}}\), find the value of \(\theta \).

attempting to use the area of sector formula (including for a semicircle)     M1

semi-circle \(\frac{1}{2}\pi  \times {5^2} = \frac{{25\pi }}{2} = 39.26990817 \ldots \)     (A1)

angle in smaller sector is \(\pi  - \theta \)     (A1)

area of sector \( = \frac{1}{2} \times {2^2} \times (\pi  - \theta )\)     (A1)

attempt to total a sum of areas of regions to 44     (M1)

\(2(\pi  - \theta ) = 44 - 39.26990817 \ldots \)

\(\theta  = 0.777{\text{ }}\left( { = \frac{{29\pi }}{4} - 22} \right)\)     A1

Note:     Award all marks except the final A1 for correct working in degrees.

Note:     Attempt to solve with goat inside triangle should lead to nonsense answer and so should only receive a maximum of the two M marks.

[6 marks]

Many students experienced difficulties with this question, mostly it seems through failing to understand the question. Some students left their answers in degrees, thereby losing the final mark.