This diagram shows a metallic pendant made out of four equal sectors of a larger circle of radius \({\text{OB}} = 9{\text{ cm}}\) and four equal sectors of a smaller circle of radius \({\text{OA}} = 3{\text{ cm}}\).
The angle \({\text{BOC}} = \) 20°.

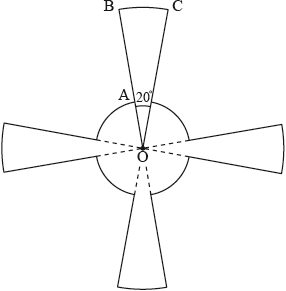
Find the area of the pendant.

METHOD 1

area = (four sector areas radius 9) + (four sector areas radius 3)     (M1)

\( = 4\left( {\frac{1}{2}{9^2}\frac{\pi }{9}} \right) + 4\left( {\frac{1}{2}{3^2}\frac{{7\pi }}{{18}}} \right)\)     (A1)(A1)

\( = 18\pi  + 7\pi \)

\( = 25\pi {\text{ }}( = 78.5{\text{ c}}{{\text{m}}^2})\)     A1

METHOD 2

area =

(area of circle radius 3) + (four sector areas radius 9) – (four sector areas radius 3)     (M1)

\(\pi {3^2} + 4\left( {\frac{1}{2}{9^2}\frac{\pi }{9}} \right) - 4\left( {\frac{1}{2}{3^2}\frac{\pi }{9}} \right)\)     (A1)(A1)

Note:     Award A1 for the second term and A1 for the third term.

\( = 9\pi  + 18\pi  - 2\pi \)

\( = 25\pi {\text{ }}( = {\text{ }}78.5{\text{ c}}{{\text{m}}^2})\)     A1

Note:     Accept working in degrees.

[4 marks]