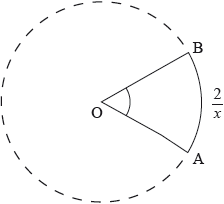
The following diagram shows a sector of a circle where ${\rm{A\hat OB}} = x$ radians and the length of the ${\text{arc AB}} = \frac{2}{x}{\text{ cm}}$.

Given that the area of the sector is $16{\text{ c}}{{\text{m}}^2}$, find the length of the arc $AB$.

${\text{arc length}} = \frac{2}{x} = rx\;\;\;\left( { \Rightarrow r = \frac{2}{{{x^2}}}} \right)$     M1

$16 = \frac{1}{2}{\left( {\frac{2}{{{x^2}}}} \right)^2}x\;\;\;\left( { \Rightarrow \frac{2}{{{x^3}}} = 16} \right)$     M1

Note:     Award M1s for attempts at the use of arc-length and sector-area formulae.

$x = \frac{1}{2}$     A1

${\text{arc length}} = {\text{4 (cm)}}$     A1

[4 marks]