| Date | May 2011 | Marks available | 2 | Reference code | 11M.2.hl.TZ1.8 |
| Level | HL | Paper | 2 | Time zone | TZ1 |
| Command term | Determine and Explain | Question number | 8 | Adapted from | N/A |
Question
Define the terms acid and base according to the Brønsted-Lowry theory. Distinguish between a weak base and a strong base. State one example of a weak base.
Weak acids in the environment may cause damage. Identify a weak acid in the environment and outline one of its effects.
The graph below indicates the pH change during the titration of 20.0 cm3 of 0.100 moldm−3 of CH3COOH(aq) with 0.100 moldm−3 KOH(aq). From the graph, identify the volume of KOH(aq) and the pH at the equivalence point.
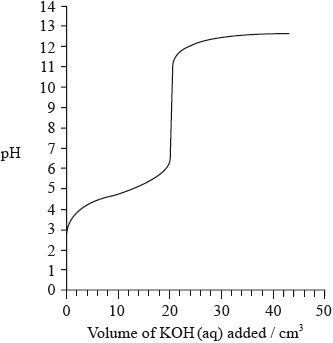
Explain how the graph could be used to determine the pKa of ethanoic acid and determine the pKa value for these data.
Sketch a graph, similar to the graph on the previous page, to indicate the change in pH during a titration of 25.0 cm3 of 0.100 moldm−3 HNO3(aq) with 0.100 moldm−3 KOH(aq). On your graph, clearly indicate the starting pH value, the equivalence point, the pH at the equivalence point and the final pH reached.
Describe how an indicator works.
Using Table 16 of the Data Booklet, identify the most appropriate indicator for the titration of ethanoic acid with potassium hydroxide. Explain your choice.
Determine the pH of the solution resulting when 100 cm3 of 0.50 moldm−3 HCl(aq) is mixed with 200 cm3 of 0.10 moldm−3 NaOH(aq).
Markscheme
Acid: proton/H+ donor and Base: proton/H+ acceptor;
Do not accept OH− for base.
Weak base: (base/electrolyte) partially dissociated/ionized (in solution/water) and Strong base: (base/electrolyte assumed to be almost) completely/100% dissociated/ionized (in solution/water) / OWTTE;
NH3/CH3CH2NH2;
Allow either name or formula or other suitable example.
sulfurous acid/H2SO3;
corrodes marble/limestone buildings/statues / leaching in soils / harms/kills
plants;
OR
nitrous acid/HNO2;
corrodes marble/limestone buildings/statues / leaching in soils / harms/kills
plants;
OR
carbonic acid/H2CO3;
corrodes marble/limestone buildings/statues / acidification of lakes;
Do not allow oxides (e.g. CO2 etc.).
Do not accept just corrodes or damages.
Volume of KOH: 20 (cm3);
Allow any value between 20 and 21 (cm3).
pH at the equivalence point: 8.0–10.0;
At half-equivalence point [CH3COOH]=[CH3COO−] so pH=pKa;
pKa=4.7;
Accept in range 4.2 to 5.2.
M2 can only be scored if M1 correct (i.e. no marks for just Data Booklet value of 4.76).

Starting pH: 1;
Equivalence point: pH=7 and 25 cm3;
Final pH reached: 12–13;
correct curve shape;
Do not award M4 if turn in curve is seen at low volumes (suggesting weak acid–strong base titration).
Award [4] if values corresponding to M1, M2 and M3 are labelled on graph (e.g using X) and correct shape of curve shown.
HIn is a weak acid / weak base;
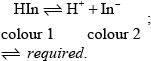
Award [2] for M2 alone.
in base equilibrium moves to right / in acid equilibrium moves to left;
phenolphthalein;
indicator colour change occurs in range of pH at the equivalence point / OWTTE;
M2 can be scored independently even if indicator is incorrect.
n(HCl)=(0.100×0.50)=0.050 (mol);
n(NaOH)=(0.200×0.10)=0.020 (mol);
n(HCl)remaining=(0.050−0.020)=0.030 (mol);
[HCl]=(0.0300.30)=0.10 (moldm−3);
pH=1.0;
Award [2 max] for just pH = 1.0 without working.
Examiners report
This was a popular question and often was well answered by candidates. In (a) (i) most candidates knew the formal definitions of an acid and a base and most could distinguish between a weak base and a strong base. Ammonia was generally given as a suitable example of a weak base. Some of the weaker students gave sodium hydroxide incorrectly as an example of a weak base which was quite surprising at HL.
In (ii), common mistakes included nitric acid and this question proved to be problematic for candidates. There were a number of G2 comments expressing some concern at asking this style of question, though this is a clear Aim 8 type question that should be explored in the formal teaching programme.
(iii) was well done.
Candidates rarely got (iv) correct.
In (v) most candidates scored either two or three, but often an incorrect shape of the curve was given.
In (b), few could describe how an indicator works and the equilibrium sign was sometimes omitted.
In (ii), phenolphthalein was usually identified as an appropriate indicator.
In (d), candidates who were able to think logically about all this did well; others scattered figures across the page and became hopelessly muddled. Often an incorrect answer of pH=7.0 was seen.

