| Date | November 2013 | Marks available | 4 | Reference code | 13N.1.sl.TZ0.2 |
| Level | SL only | Paper | 1 | Time zone | TZ0 |
| Command term | Complete | Question number | 2 | Adapted from | N/A |
Question
UU is the set of positive integers less than or equal to 1010.
AA, BB and CC are subsets of UU.
A={even integers}A={even integers}
B={multiples of 3}B={multiples of 3}
C={6, 7, 8, 9}C={6, 7, 8, 9}
List the elements of AA.
List the elements of BB.
Complete the Venn diagram with all the elements of UU.
Markscheme
2,4,6,8,102,4,6,8,10 (A1) (C1)
Note: Do not penalize the use of { }{ }.
[1 mark]
3,6,93,6,9 (A1) (C1)
Note: Do not penalize the use of { }{ }.
Follow through from part (a) only if their UU is listed.
[1 mark]
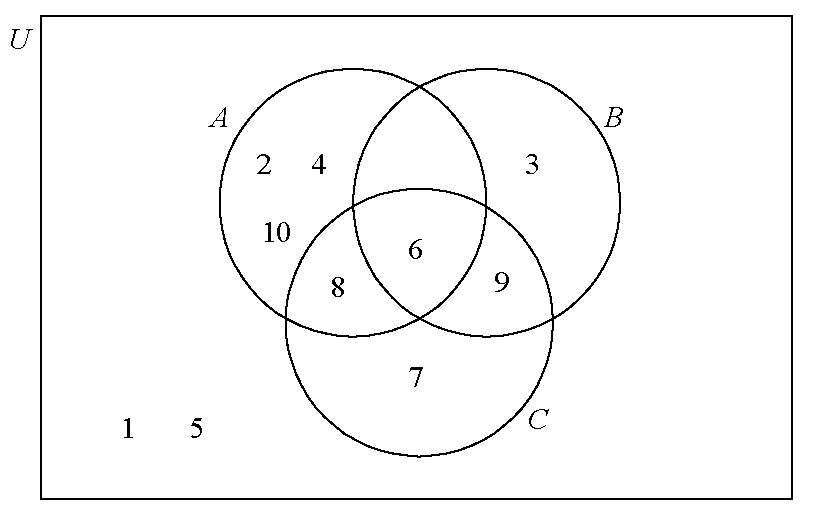(A1)(ft)(A1)(ft)(A1)(ft)(A1)(ft) (C4)
Notes: Award (A1)(ft) for the correct placement of 66.
Award (A1)(ft) for the correct placement of 88 and 99 and the empty region.
Award (A1)(ft) for the correct placement of 22, 44, 33, 77, and 1010.
Award (A1)(ft) for the correct placement of 11 and 55.
If an element is in more than one region, award (A0) for that element.
Follow through from their answers to parts (a) and (b).
[4 marks]
Examiners report
This question was done well by most candidates. The most frequent error was to omit the placement of 1 and 5 or to include 0 in the set of even integers.
This question was done well by most candidates. The most frequent error was to omit the placement of 1 and 5 or to include 0 in the set of even integers.
This question was done well by most candidates. The most frequent error was to omit the placement of 1 and 5 or to include 0 in the set of even integers.
