| Date | May 2010 | Marks available | 3 | Reference code | 10M.1.sl.TZ1.9 |
| Level | SL only | Paper | 1 | Time zone | TZ1 |
| Command term | Find | Question number | 9 | Adapted from | N/A |
Question
Let f(x)=cosxsinxf(x)=cosxsinx , for sinx≠0sinx≠0 .
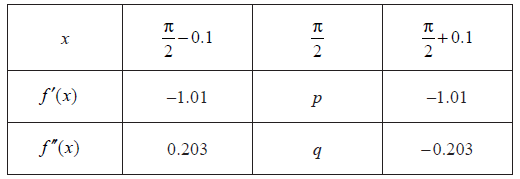
In the following table, f′(π2)=p and f″(π2)=q . The table also gives approximate values of f′(x) and f″(x) near x=π2 .
Use the quotient rule to show that f′(x)=−1sin2x .
Find f″(x) .
Find the value of p and of q.
Use information from the table to explain why there is a point of inflexion on the graph of f where x=π2 .
Markscheme
ddxsinx=cosx , ddxcosx=−sinx (seen anywhere) (A1)(A1)
evidence of using the quotient rule M1
correct substitution A1
e.g. sinx(−sinx)−cosx(cosx)sin2x , −sin2x−cos2xsin2x
f′(x)=−(sin2x+cos2x)sin2x A1
f′(x)=−1sin2x AG N0
[5 marks]
METHOD 1
appropriate approach (M1)
e.g. f′(x)=−(sinx)−2
f″(x)=2(sin−3x)(cosx) (=2cosxsin3x) A1A1 N3
Note: Award A1 for 2sin−3x , A1 for cosx .
METHOD 2
derivative of sin2x=2sinxcosx (seen anywhere) A1
evidence of choosing quotient rule (M1)
e.g. u=−1 , v=sin2x , f″=sin2x×0−(−1)2sinxcosx(sin2x)2
f″(x)=2sinxcosx(sin2x)2 (=2cosxsin3x) A1 N3
[3 marks]
evidence of substituting π2 M1
e.g. −1sin2π2 , 2cosπ2sin3π2
p=−1 , q=0 A1A1 N1N1
[3 marks]
second derivative is zero, second derivative changes sign R1R1 N2
[2 marks]
Examiners report
Many candidates comfortably applied the quotient rule, although some did not completely show that the Pythagorean identity achieves the numerator of the answer given. Whether changing to −(sinx)−2 , or applying the quotient rule a second time, most candidates neglected the chain rule in finding the second derivative.
Whether changing to −(sinx)−2 , or applying the quotient rule a second time, most candidates neglected the chain rule in finding the second derivative.
Those who knew the trigonometric ratios at π2typically found the values of p and of q, sometimes in follow-through from an incorrect f″(x) .
Few candidates gave two reasons from the table that supported the existence of a point of inflexion. Most stated that the second derivative is zero and neglected to consider the sign change to the left and right of q. Some discussed a change of concavity, but without supporting this statement by referencing the change of sign in f″(x) , so no marks were earned.

