| Date | November 2016 | Marks available | 2 | Reference code | 16N.2.sl.TZ0.1 |
| Level | SL only | Paper | 2 | Time zone | TZ0 |
| Command term | Find | Question number | 1 | Adapted from | N/A |
Question
Let \(f(x) = {x^2} + 2x + 1\) and \(g(x) = x - 5\), for \(x \in \mathbb{R}\).
Find \(f(8)\).
[2]
a.
Find \((g \circ f)(x)\).
[2]
b.
Solve \((g \circ f)(x) = 0\).
[3]
c.
Markscheme
attempt to substitute \(x = 8\) (M1)
eg\(\,\,\,\,\,\)\({8^2} + 2 \times 8 + 1\)
\(f(8) = 81\) A1 N2
[2 marks]
a.
attempt to form composition (in any order) (M1)
eg\(\,\,\,\,\,\)\(f(x - 5),{\text{ }}g\left( {f(x)} \right),{\text{ }}\left( {{x^2} + 2x + 1} \right) - 5\)
\((g \circ f)(x) = {x^2} + 2x - 4\) A1 N2
[2 marks]
b.
valid approach (M1)
eg \(x = \frac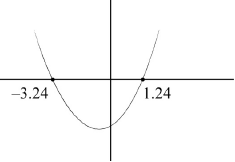{{ - 2 \pm \sqrt {20} }}{2}\), 
\(1.23606,{\text{ }} - 3.23606\)
\(x = 1.24,{\text{ }}x = - 3.24\) A1A1 N3
[3 marks]
c.
Examiners report
[N/A]
a.
[N/A]
b.
[N/A]
c.
