DP Mathematics: Analysis and Approaches Questionbank

SL 4.5—Probability concepts, expected numbers
Description
[N/A]Directly related questions
-
20N.1.SL.TZ0.S_8a:
Find the value of a.
-
20N.1.SL.TZ0.S_8b:
Write down the value of the median distance in kilometres (km).
-
20N.1.SL.TZ0.S_8c:
Find the value of b.
-
20N.1.SL.TZ0.S_8d:
Find m.
-
20N.1.SL.TZ0.S_8e:
The first 150 athletes that completed the race won a prize.
Given that an athlete took between 22 and m minutes to complete the 5 km race, calculate the probability that they won a prize.
-
20N.2.SL.TZ0.S_9a:
Find the probability that it will take Fiona between 15 minutes and 30 minutes to walk to the bus stop.
-
20N.2.SL.TZ0.S_9b:
Find σ.
-
20N.2.SL.TZ0.S_9c:
Find the probability that the bus journey takes less than 45 minutes.
-
20N.2.SL.TZ0.S_9d:
Find the probability that Fiona will arrive on time.
-
20N.2.SL.TZ0.S_9e:
This year, Fiona will go to school on 183 days.
Calculate the number of days Fiona is expected to arrive on time.
-
20N.1.SL.TZ0.T_6a.i:
Find the probability that the first ball chosen is labelled A.
-
20N.1.SL.TZ0.T_6a.ii:
Find the probability that the first ball chosen is labelled A or labelled N.
-
20N.1.SL.TZ0.T_6b:
Find the probability that the second ball chosen is labelled A, given that the first ball chosen was labelled N.
-
20N.1.SL.TZ0.T_6c:
Find the probability that both balls chosen are labelled N.
-
22M.1.SL.TZ2.9c:
Assuming that rolls of the die are independent, find the probability that Nicky wins the game.
-
19M.2.SL.TZ1.S_10a.i:
Find the probability of rolling exactly one red face.
-
19M.2.SL.TZ1.S_10a.ii:
Find the probability of rolling two or more red faces.
-
19M.2.SL.TZ1.S_10b:
Show that, after a turn, the probability that Ted adds exactly $10 to his winnings is 13.
-
19M.2.SL.TZ1.S_10c.i:
Write down the value of x.
-
19M.2.SL.TZ1.S_10c.ii:
Hence, find the value of y.
-
19M.2.SL.TZ1.S_10d:
Ted will always have another turn if he expects an increase to his winnings.
Find the least value of w for which Ted should end the game instead of having another turn.
-
17M.1.SL.TZ1.S_1a.i:
Find the value of p;
-
17M.1.SL.TZ1.S_1a.ii:
Find the value of q.
-
17M.1.SL.TZ1.S_1b:
A girl is selected at random. Find the probability that she takes economics but not history.
-
18N.1.SL.TZ0.S_9a.i:
Find the probability, in terms of n, that the game will end on her first draw.
-
18N.1.SL.TZ0.S_9a.ii:
Find the probability, in terms of n, that the game will end on her second draw.
-
18N.1.SL.TZ0.S_9b.i:
third draw.
-
18N.1.SL.TZ0.S_9b.ii:
fourth draw.
-
18N.1.SL.TZ0.S_9c:
Hayley plays the game when n = 5. She pays $20 to play and can earn money back depending on the number of draws it takes to obtain a blue marble. She earns no money back if she obtains a blue marble on her first draw. Let M be the amount of money that she earns back playing the game. This information is shown in the following table.
Find the value of k so that this is a fair game.
-
18N.2.SL.TZ0.S_1a:
Write down the number of students in the group who take art class.
-
18N.2.SL.TZ0.S_1b.i:
the student does not take art class.
-
18N.2.SL.TZ0.S_1b.ii:
the student takes either art class or music class, but not both.
-
17N.1.SL.TZ0.T_7a:
Complete the Venn diagram for these students.
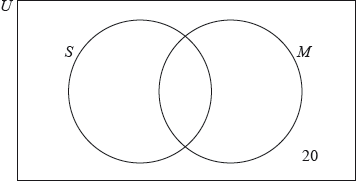
-
17N.1.SL.TZ0.T_7b:
One of the students who joined the sports club is chosen at random. Find the probability that this student joined both clubs.
-
17N.1.SL.TZ0.T_7c:
Determine whether the events S and M are independent.
-
16N.2.SL.TZ0.T_2a:
Draw a Venn diagram to represent the given information, using sets labelled B, C and H.
-
16N.2.SL.TZ0.T_2b:
Show that x=3.
-
16N.2.SL.TZ0.T_2c:
Write down the value of n(B∩C).
-
16N.2.SL.TZ0.T_2d:
Find the probability that this person
(i) went on at most one trip;
(ii) went on the coach trip, given that this person also went on both the helicopter trip and the boat trip.
-
18N.2.SL.TZ0.T_2a.i:
Find the number of students in the school that are taught in Spanish.
-
18N.2.SL.TZ0.T_2a.ii:
Find the number of students in the school that study Mathematics in English.
-
18N.2.SL.TZ0.T_2a.iii:
Find the number of students in the school that study both Biology and Mathematics.
-
18N.2.SL.TZ0.T_2b.i:
Write down n(S∩(M∪B)).
-
18N.2.SL.TZ0.T_2b.ii:
Write down n(B∩M∩S′).
-
18N.2.SL.TZ0.T_2c.i:
Find the probability that this student studies Mathematics.
-
18N.2.SL.TZ0.T_2c.ii:
Find the probability that this student studies neither Biology nor Mathematics.
-
18N.2.SL.TZ0.T_2c.iii:
Find the probability that this student is taught in Spanish, given that the student studies Biology.
-
19M.1.SL.TZ2.T_5a:
Using the given information, complete the following Venn diagram.
-
19M.1.SL.TZ2.T_5b:
Find the number of surveyed students who did not like any of the three flavours.
-
19M.1.SL.TZ2.T_5c:
A student is chosen at random from the surveyed students.
Find the probability that this student likes kiwi fruit smoothies given that they like mango smoothies.
-
16N.2.SL.TZ0.T_6a:
Write down a formula for A, the surface area to be coated.
-
16N.2.SL.TZ0.T_6b:
Express this volume in cm3.
-
16N.2.SL.TZ0.T_6c:
Write down, in terms of r and h, an equation for the volume of this water container.
-
16N.2.SL.TZ0.T_6d:
Show that A=πr2+1000000r.
-
16N.2.SL.TZ0.T_6e:
Find dAdr.
-
16N.2.SL.TZ0.T_6f:
Using your answer to part (e), find the value of r which minimizes A.
-
16N.2.SL.TZ0.T_6g:
Find the value of this minimum area.
-
16N.2.SL.TZ0.T_6h:
Find the least number of cans of water-resistant material that will coat the area in part (g).
