| Date | May 2017 | Marks available | 3 | Reference code | 17M.1.AHL.TZ1.H_12 |
| Level | Additional Higher Level | Paper | Paper 1 (without calculator) | Time zone | Time zone 1 |
| Command term | Show that | Question number | H_12 | Adapted from | N/A |
Question
Consider the function q(x)=x5−10x2+15x−6, x∈R.
Show that the graph of y=q(x) is concave up for x>1.
[3]
e.i.
Sketch the graph of y=q(x) showing clearly any intercepts with the axes.
[3]
e.ii.
Markscheme
d2ydx2=20x3−20 M1A1
for x>1, 20x3−20>0⇒ concave up R1AG
[3 marks]
e.i.
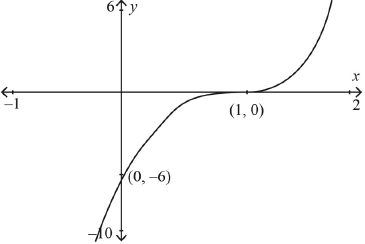
x-intercept at (1, 0) A1
y-intercept at (0, −6) A1
stationary point of inflexion at (1, 0) with correct curvature either side A1
[3 marks]
e.ii.
Examiners report
[N/A]
e.i.
[N/A]
e.ii.

