DP Physics Questionbank

Option D: Astrophysics (Core topics)
Description
Overview of the essential ideas for this topic
D.1: One of the most difficult problems in astronomy is coming to terms with the vast distances between stars and galaxies and devising accurate methods for measuring them.
D.2: A simple diagram that plots the luminosity versus the surface temperature of stars reveals unusually detailed patterns that help understand the inner workings of stars. Stars follow well-defined patterns from the moment they are created out of collapsing interstellar gas, to their lives on the main sequence and to their eventual death.
D.3: The Hot Big Bang model is a theory that describes the origin and expansion of the universe and is supported by extensive experimental evidence.
Directly related questions
-
16N.3.SL.TZ0.15e:
A standard Hertzsprung–Russell (HR) diagram is shown.
Using the HR diagram, draw the present position of Alpha Centauri A and its expected evolutionary path.
-
16N.3.SL.TZ0.15b:
(i) Calculate bAbB=apparent brightness of Alpha Centauri Aapparent brightness of Alpha Centauri B.
(ii) The luminosity of the Sun is 3.8 × 1026 W. Calculate the radius of Alpha Centauri A.
-
16N.3.SL.TZ0.16a:
Determine the distance from Earth to the Cepheid star in parsecs. The luminosity of the Sun is 3.8 × 1026 W. The average apparent brightness of the Cepheid star is 1.1 × 10–9 W m–2.
- 16N.3.SL.TZ0.17b: A spectral line in the hydrogen spectrum measured in the laboratory today has a wavelength of...
- 16N.3.SL.TZ0.15a: State what is meant by a binary star system.
- 16N.3.SL.TZ0.15d: Alpha Centauri A is in equilibrium at constant radius. Explain how this equilibrium is maintained.
-
16N.3.SL.TZ0.15c:
Show, without calculation, that the radius of Alpha Centauri B is smaller than the radius of Alpha Centauri A.
- 16N.3.SL.TZ0.17a: Identify two other characteristics of the CMB radiation that are predicted from the Hot Big Bang...
- 16N.3.SL.TZ0.16b: Explain why Cephids are used as standard candles.
-
17M.3.SL.TZ1.9a.iv:
Determine the distance of Theta 1 Orionis in AU.
-
17M.3.SL.TZ1.10c.ii:
Estimate the size of the Universe relative to its present size when the light was emitted by the galaxy in (c).
-
17M.3.SL.TZ1.9a.i:
State what is meant by a main sequence star.
-
17M.3.SL.TZ1.10a.i:
State two characteristics of the cosmic microwave background (CMB) radiation.
-
17M.3.SL.TZ1.10c.i:
Determine the distance to this galaxy using a value for the Hubble constant of H0 = 68 km s–1Mpc–1.
-
17M.3.SL.TZ1.10a.ii:
The present temperature of the CMB is 2.8 K. Calculate the peak wavelength of the CMB.
-
17M.3.SL.TZ1.9a.ii:
Show that the mass of Theta 1 Orionis is about 40 solar masses.
-
17M.3.SL.TZ1.9b:
Discuss how Theta 1 Orionis does not collapse under its own weight.
-
17M.3.SL.TZ1.10b:
Describe how the CMB provides evidence for the Hot Big Bang model of the universe.
-
17M.3.SL.TZ1.9a.iii:
The surface temperature of the Sun is about 6000 K. Estimate the surface temperature of Theta 1 Orionis.
-
17M.3.SL.TZ1.9c:
The Sun and Theta 1 Orionis will eventually leave the main sequence. Compare and contrast the different stages in the evolution of the two stars.
-
17M.3.SL.TZ2.11b:
The Hertzsprung–Russell (HR) diagram shows two main sequence stars X and Y and includes lines of constant radius. R is the radius of the Sun.
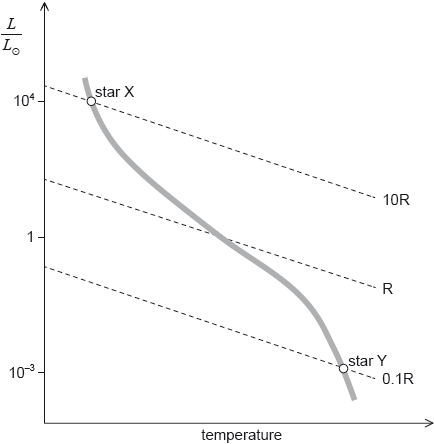
Using the mass–luminosity relation and information from the graph, determine the ratio density of star Xdensity of star Y.
-
17M.3.SL.TZ2.11c.iii:
The radius of a typical neutron star is 20 km and its surface temperature is 106 K. Determine the luminosity of this neutron star.
-
17M.3.SL.TZ2.11a:
State the most abundant element in the core and the most abundant element in the outer layer.
-
17M.3.SL.TZ2.11c.iv:
Determine the region of the electromagnetic spectrum in which the neutron star in (c)(iii) emits most of its energy.
-
17M.3.SL.TZ2.12b:
State two features of the cosmic microwave background (CMB) radiation which are consistent with the Big Bang model.
-
17M.3.SL.TZ2.12a:
Describe what is meant by the Big Bang model of the universe.
-
17M.3.SL.TZ2.11c.ii:
Outline why the neutron star that is left after the supernova stage does not collapse under the action of gravitation.
-
17M.3.SL.TZ2.11c.i:
On the HR diagram in (b), draw a line to indicate the evolutionary path of star X.
-
17M.3.SL.TZ2.12c.ii:
Describe how type Ia supernovae could be used to measure the distance to this galaxy.
-
17M.3.SL.TZ2.12c.i:
Determine the distance to the galaxy in Mpc.
-
20N.3.SL.TZ0.17a:
Show by calculation that Eta Aquilae A is not on the main sequence.
- 20N.3.SL.TZ0.17d: Eta Aquilae A is a Cepheid variable. Explain why the brightness of Eta Aquilae A varies.
-
20N.3.SL.TZ0.16b:
Outline how Hubble’s law is related to z.
- 20N.3.SL.TZ0.14b(i): Comets develop a tail as they approach the Sun. Identify one other characteristic of comets.
-
20N.3.SL.TZ0.15a:
Show that the apparent brightness b∝AT4d2, where d is the distance of the object from Earth, T is the surface temperature of the object and A is the surface area of the object.
-
20N.3.SL.TZ0.16a:
The light from a distant galaxy shows that z=0.11.
Calculate the ratio size of the universe when the light was emittedsize of the universe at present.
-
20N.3.SL.TZ0.14a:
The astronomical unit (AU) and light year (ly) are convenient measures of distance in astrophysics. Define each unit.
AU:
ly:
-
20N.3.SL.TZ0.15b:
Two of the brightest objects in the night sky seen from Earth are the planet Venus and the star Sirius. Explain why the equation b∝AT4d2 is applicable to Sirius but not to Venus.
-
20N.3.SL.TZ0.17b(i):
Estimate, in pc, the distance to Eta Aquilae A using the parallax angle in the table.
-
20N.3.HL.TZ0.21b:
Outline how Hubble’s law is related to z.
- 20N.3.SL.TZ0.14b(ii): Identify one object visible in the image that is outside our Solar System.
- 20N.3.HL.TZ0.22d: Eta Aquilae A is a Cepheid variable. Explain why the brightness of Eta Aquilae A varies.
-
20N.3.SL.TZ0.17b(ii):
Estimate, in pc, the distance to Eta Aquilae A using the luminosity in the table, given that L⊙=3.83×1026 W.
-
20N.3.HL.TZ0.22b(ii):
Estimate, in pc, the distance to Eta Aquilae A using the luminosity in the table, given that L⊙=3.83×1026 W.
-
20N.3.HL.TZ0.22b(i):
Estimate, in pc, the distance to Eta Aquilae A using the parallax angle in the table.
-
20N.3.HL.TZ0.21a:
The light from a distant galaxy shows that z=0.11.
Calculate the ratio size of the universe when the light was emittedsize of the universe at present.
-
20N.3.HL.TZ0.22a:
Show by calculation that Eta Aquilae A is not on the main sequence.
-
17N.3.SL.TZ0.13b:
Determine the velocity of the galaxy relative to Earth.
-
17N.3.SL.TZ0.12d.i:
Determine the radius of Sirius B in terms of the radius of the Sun.
- 17N.3.SL.TZ0.12d.ii: Identify the star type of Sirius B.
- 17N.3.SL.TZ0.12e.i: draw the approximate positions of Sirius A, labelled A and Sirius B, labelled B.
- 17N.3.SL.TZ0.12e.ii: sketch the expected evolutionary path for Sirius A.
- 17N.3.SL.TZ0.13a: Outline one reason for the difference in wavelength.
-
18M.3.SL.TZ2.11d.ii:
plot the position, using the letter P, of the main sequence star P you calculated in (b).
-
18M.3.SL.TZ2.11d.iii:
plot the position, using the letter G, of Gacrux.
-
18M.3.SL.TZ2.11c.i:
The luminosity of the Sun L⊙ is 3.85 × 1026 W. Determine the luminosity of Gacrux relative to the Sun.
-
18M.3.SL.TZ2.11c.ii:
The distance to Gacrux can be determined using stellar parallax. Outline why this method is not suitable for all stars.
-
18M.3.SL.TZ2.12a:
Estimate, using the data, the age of the universe. Give your answer in seconds.
-
18M.3.SL.TZ2.11a:
Main sequence stars are in equilibrium under the action of forces. Outline how this equilibrium is achieved.
-
18M.3.SL.TZ2.11e:
Discuss, with reference to its change in mass, the evolution of star P from the main sequence until its final stable phase.
-
18M.3.SL.TZ2.11b:
A main sequence star P, is 1.3 times the mass of the Sun. Calculate the luminosity of P relative to the Sun.
-
18M.3.SL.TZ2.11d.i:
draw the main sequence.
-
18M.3.SL.TZ2.12b:
Identify the assumption that you made in your answer to (a).
-
18M.3.SL.TZ2.12c:
On the graph, one galaxy is labelled A. Determine the size of the universe, relative to its present size, when light from the galaxy labelled A was emitted.
-
18M.3.SL.TZ1.10a.i:
Distinguish between the solar system and a galaxy.
-
18M.3.SL.TZ1.10a.ii:
Distinguish between a planet and a comet.
-
18M.3.SL.TZ1.11a.i:
Suggest, using the graphs, why star X is most likely to be a main sequence star.
-
18M.3.SL.TZ1.11a.ii:
Show that the temperature of star X is approximately 10 000 K.
-
18M.3.SL.TZ1.11b.i:
Write down the luminosity of star X (LX) in terms of the luminosity of the Sun (Ls).
-
18M.3.SL.TZ1.11b.ii:
Determine the radius of star X (RX) in terms of the radius of the Sun (Rs).
-
18M.3.SL.TZ1.11b.iii:
Estimate the mass of star X (MX) in terms of the mass of the Sun (Ms).
-
18M.3.SL.TZ1.11c:
Star X is likely to evolve into a stable white dwarf star.
Outline why the radius of a white dwarf star reaches a stable value.
-
18M.3.SL.TZ1.12a:
Explain how international collaboration has helped to refine this value.
-
18M.3.SL.TZ1.12b:
Estimate, in Mpc, the distance between the galaxy and the Earth.
- 21N.1.SL.TZ0.30: Which is correct for a black-body radiator? A. The power it emits from a unit surface area...
- 18N.3.SL.TZ0.12d: Describe the stages in the evolution of Epsilon Indi from the point when it leaves the main...
-
18N.3.SL.TZ0.12b:
Epsilon Indi is a main sequence star. Show that the mass of Epsilon Indi is 0.64 M⊙.
- 18N.3.SL.TZ0.12a.ii: Using the axis, draw the variation with wavelength of the intensity of the radiation emitted by...
-
18N.3.HL.TZ0.18a.i:
Determine the peak wavelength of the radiation emitted by Epsilon Indi.
- 18N.3.SL.TZ0.12c: Describe how the chemical composition of a star may be determined.
-
18N.3.HL.TZ0.19b:
Use the graph to determine the age of the universe in s.
- 18N.3.SL.TZ0.11a: Distinguish between a constellation and an open cluster.
-
18N.3.SL.TZ0.13a:
Outline how Hubble measured the recessional velocities of galaxies.
- 18N.3.SL.TZ0.11b.ii: State why there is a maximum distance that astronomers can measure using stellar parallax.
-
18N.3.SL.TZ0.12a.iii:
The following data are available for the Sun.
Surface temperature = 5800 K
Luminosity = L⊙
Mass = M⊙
Radius = R⊙
Epsilon Indi has a radius of 0.73 R⊙. Show that the luminosity of Epsilon Indi is 0.2 L⊙.
- 18N.3.SL.TZ0.11b.i: The parallax angle of Mintaka measured from Earth is 3.64 × 10–3 arc-second. Calculate, in...
-
18N.3.SL.TZ0.12a.i:
Determine the peak wavelength of the radiation emitted by Epsilon Indi.
-
18N.3.SL.TZ0.13b:
Using the graph, determine in s, the age of the universe.
- 18N.3.HL.TZ0.17b.ii: State why there is a maximum distance that astronomers can measure using stellar parallax.
- 18N.3.HL.TZ0.17b.i: The parallax angle of Mintaka measured from Earth is 3.64 × 10–3 arc-second. Calculate, in...
-
18N.3.HL.TZ0.19a:
Outline how Hubble measured the recessional velocities of galaxies.
- 18N.3.HL.TZ0.17a: Distinguish between a constellation and an open cluster.
-
18N.3.HL.TZ0.18b:
Epsilon Indi is a main sequence star. Show that the mass of Epsilon Indi is 0.64 M⊙.
-
18N.3.HL.TZ0.18a.iii:
The following data are available for the Sun.
Surface temperature = 5800 K
Luminosity = L⊙
Mass = M⊙
Radius = R⊙
Epsilon Indi has a radius of 0.73 R⊙. Show that the luminosity of Epsilon Indi is 0.2 L⊙.
- 18N.3.HL.TZ0.18a.ii: Using the axis, draw the variation with wavelength of the intensity of the radiation emitted by...
- 18N.3.HL.TZ0.18d: Describe the stages in the evolution of Epsilon Indi from the point when it leaves the main...
-
18N.3.HL.TZ0.18c:
The Sun will spend about nine billion years on the main sequence. Calculate how long Epsilon Indi will spend on the main sequence.
- 19M.3.SL.TZ2.14ai: A galaxy is 1.6 × 108 ly from Earth. Show that its recessional speed as measured from Earth is...
- 19M.3.SL.TZ2.13aii: Explain how Cepheid variables are used to determine distances.
-
19M.3.SL.TZ2.14aii:
A line in the hydrogen spectrum when measured on Earth has a wavelength of 486 nm. Calculate, in nm, the wavelength of the same hydrogen line when observed in the galaxy’s emission spectrum.
- 19M.3.SL.TZ2.15b: Suggest the conditions that will cause the Sun to become a red giant.
-
19M.3.SL.TZ2.13bi:
Determine the peak apparent brightness of δ-Cephei as observed from Earth.
-
19M.3.SL.TZ2.13bii:
Calculate the peak surface temperature of δ-Cephei.
- 19M.3.SL.TZ2.13ai: Outline the processes that produce the change of luminosity with time of Cepheid variables.
- 19M.3.SL.TZ2.14b: Outline how observations of spectra from distant galaxies provide evidence that the universe is...
-
19M.3.SL.TZ2.15c:
Outline why the Sun will maintain a constant radius after it becomes a white dwarf.
- 19M.3.SL.TZ2.15a: Identify, on the HR diagram, the position of the Sun. Label the position S.
- 19M.3.HL.TZ1.19b: Suggest why type I a supernovae were used in the study that led to the conclusion that the...
-
19M.3.SL.TZ2.15d:
During its evolution, the Sun is likely to be a red giant of surface temperature 3000 K and luminosity 104 L☉. Later it is likely to be a white dwarf of surface temperature 10 000 K and luminosity 10-4 L☉. Calculate the radius of the Sun as a white dwarfradius of the Sun as a red giant.
-
17N.3.SL.TZ0.12c:
The mass of Sirius B is about the same mass as the Sun. The luminosity of Sirius B is 2.5 % of the luminosity of the Sun. Show, with a calculation, that Sirius B is not a main sequence star.
-
17N.3.SL.TZ0.12b:
The peak spectral line of Sirius B has a measured wavelength of 115 nm. Show that the surface temperature of Sirius B is about 25 000 K.
- 17N.3.SL.TZ0.12a: State what is meant by a binary star.
-
19N.3.SL.TZ0.10b(ii):
The surface temperature of Eta Cassiopeiae B is 4100 K. Determine the ratio radius of the Eta Cassiopeiae Aradius of the Eta Cassiopeiae B.
-
19N.3.SL.TZ0.10c(iii):
Calculate the ratio mass of Eta Cassiopeiae Amass of the Sun.
-
19N.3.SL.TZ0.10b(i):
The peak wavelength of radiation from Eta Cassiopeiae A is 490 nm. Show that the surface temperature of Eta Cassiopeiae A is about 6000 K.
-
19N.3.SL.TZ0.11b(i):
Estimate the age of the universe in seconds using the Hubble constant H0 = 70 km s–1 Mpc–1.
- 19N.3.SL.TZ0.10 a: Distinguish between a constellation and a stellar cluster.
- 19N.3.SL.TZ0.10c(i): On the HR diagram, draw the present position of Eta Cassiopeiae A.
- 19N.3.SL.TZ0.10c(ii): State the star type of Eta Cassiopeiae A.
-
19N.3.SL.TZ0.10c(iv):
Deduce the final evolutionary state of Eta Cassiopeiae A.
-
19N.3.SL.TZ0.10b(iii):
The distance of the Eta Cassiopeiae system from the Earth is 1.8 × 1017 m. Calculate, in terms of L⊙, the luminosity of Eta Cassiopeiae A.
- 19N.3.SL.TZ0.11a(i): Outline how the light spectra of distant galaxies are used to confirm hypotheses about the...
-
19N.3.SL.TZ0.11b(ii):
Outline why the estimate made in (b)(i) is unlikely to be the actual age of the universe.
-
19N.3.SL.TZ0.11a(ii):
Light from a hydrogen source in a laboratory on Earth contains a spectral line of wavelength 122 nm. Light from the same spectral line reaching Earth from a distant galaxy has a wavelength of 392 nm. Determine the ratio of the present size of the universe to the size of the universe when the light was emitted by the galaxy.
- 19N.3.HL.TZ0.16a(i): Outline how the light spectra of distant galaxies are used to confirm hypotheses about the...
-
19N.3.HL.TZ0.16a(ii):
Light from a hydrogen source in a laboratory on Earth contains a spectral line of wavelength 122 nm. Light from the same spectral line reaching Earth from a distant galaxy has a wavelength of 392 nm. Determine the ratio of the present size of the universe to the size of the universe when the light was emitted by the galaxy.
- 19N.3.SL.TZ0.10a: Distinguish between a constellation and a stellar cluster.
Sub sections and their related questions
D.1 – Stellar quantities
- 16N.3.SL.TZ0.15a: State what is meant by a binary star system.
-
16N.3.SL.TZ0.15b:
(i) Calculate bAbB=apparent brightness of Alpha Centauri Aapparent brightness of Alpha Centauri B.
(ii) The luminosity of the Sun is 3.8 × 1026 W. Calculate the radius of Alpha Centauri A.
-
17M.3.SL.TZ1.9a.i:
State what is meant by a main sequence star.
-
17M.3.SL.TZ1.9a.iii:
The surface temperature of the Sun is about 6000 K. Estimate the surface temperature of Theta 1 Orionis.
-
17M.3.SL.TZ1.9a.iv:
Determine the distance of Theta 1 Orionis in AU.
-
17M.3.SL.TZ1.9b:
Discuss how Theta 1 Orionis does not collapse under its own weight.
-
17M.3.SL.TZ2.11a:
State the most abundant element in the core and the most abundant element in the outer layer.
-
17M.3.SL.TZ2.11c.iii:
The radius of a typical neutron star is 20 km and its surface temperature is 106 K. Determine the luminosity of this neutron star.
-
18M.3.SL.TZ1.10a.i:
Distinguish between the solar system and a galaxy.
-
18M.3.SL.TZ1.10a.ii:
Distinguish between a planet and a comet.
-
18M.3.SL.TZ2.11a:
Main sequence stars are in equilibrium under the action of forces. Outline how this equilibrium is achieved.
-
18M.3.SL.TZ2.11b:
A main sequence star P, is 1.3 times the mass of the Sun. Calculate the luminosity of P relative to the Sun.
-
18M.3.SL.TZ2.11c.i:
The luminosity of the Sun L⊙ is 3.85 × 1026 W. Determine the luminosity of Gacrux relative to the Sun.
-
18M.3.SL.TZ2.11c.ii:
The distance to Gacrux can be determined using stellar parallax. Outline why this method is not suitable for all stars.
- 18N.3.SL.TZ0.11a: Distinguish between a constellation and an open cluster.
- 18N.3.SL.TZ0.11b.i: The parallax angle of Mintaka measured from Earth is 3.64 × 10–3 arc-second. Calculate, in...
- 18N.3.SL.TZ0.11b.ii: State why there is a maximum distance that astronomers can measure using stellar parallax.
-
18N.3.SL.TZ0.12a.iii:
The following data are available for the Sun.
Surface temperature = 5800 K
Luminosity = L⊙
Mass = M⊙
Radius = R⊙
Epsilon Indi has a radius of 0.73 R⊙. Show that the luminosity of Epsilon Indi is 0.2 L⊙.
- 18N.3.HL.TZ0.17a: Distinguish between a constellation and an open cluster.
- 18N.3.HL.TZ0.17b.i: The parallax angle of Mintaka measured from Earth is 3.64 × 10–3 arc-second. Calculate, in...
- 18N.3.HL.TZ0.17b.ii: State why there is a maximum distance that astronomers can measure using stellar parallax.
-
18N.3.HL.TZ0.18a.iii:
The following data are available for the Sun.
Surface temperature = 5800 K
Luminosity = L⊙
Mass = M⊙
Radius = R⊙
Epsilon Indi has a radius of 0.73 R⊙. Show that the luminosity of Epsilon Indi is 0.2 L⊙.
-
19M.3.SL.TZ2.13bi:
Determine the peak apparent brightness of δ-Cephei as observed from Earth.
-
19M.3.SL.TZ2.15d:
During its evolution, the Sun is likely to be a red giant of surface temperature 3000 K and luminosity 104 L☉. Later it is likely to be a white dwarf of surface temperature 10 000 K and luminosity 10-4 L☉. Calculate the radius of the Sun as a white dwarfradius of the Sun as a red giant.
- 19N.3.SL.TZ0.10 a: Distinguish between a constellation and a stellar cluster.
-
19N.3.SL.TZ0.10b(ii):
The surface temperature of Eta Cassiopeiae B is 4100 K. Determine the ratio radius of the Eta Cassiopeiae Aradius of the Eta Cassiopeiae B.
-
19N.3.SL.TZ0.10b(iii):
The distance of the Eta Cassiopeiae system from the Earth is 1.8 × 1017 m. Calculate, in terms of L⊙, the luminosity of Eta Cassiopeiae A.
- 19N.3.SL.TZ0.10a: Distinguish between a constellation and a stellar cluster.
-
20N.3.SL.TZ0.14a:
The astronomical unit (AU) and light year (ly) are convenient measures of distance in astrophysics. Define each unit.
AU:
ly:
- 20N.3.SL.TZ0.14b(i): Comets develop a tail as they approach the Sun. Identify one other characteristic of comets.
- 20N.3.SL.TZ0.14b(ii): Identify one object visible in the image that is outside our Solar System.
-
20N.3.SL.TZ0.15a:
Show that the apparent brightness b∝AT4d2, where d is the distance of the object from Earth, T is the surface temperature of the object and A is the surface area of the object.
-
20N.3.SL.TZ0.15b:
Two of the brightest objects in the night sky seen from Earth are the planet Venus and the star Sirius. Explain why the equation b∝AT4d2 is applicable to Sirius but not to Venus.
-
20N.3.SL.TZ0.17b(i):
Estimate, in pc, the distance to Eta Aquilae A using the parallax angle in the table.
-
20N.3.SL.TZ0.17b(ii):
Estimate, in pc, the distance to Eta Aquilae A using the luminosity in the table, given that L⊙=3.83×1026 W.
-
20N.3.HL.TZ0.22b(i):
Estimate, in pc, the distance to Eta Aquilae A using the parallax angle in the table.
-
20N.3.HL.TZ0.22b(ii):
Estimate, in pc, the distance to Eta Aquilae A using the luminosity in the table, given that L⊙=3.83×1026 W.
- 21N.1.SL.TZ0.30: Which is correct for a black-body radiator? A. The power it emits from a unit surface area...
D.2 – Stellar characteristics and stellar evolution
-
16N.3.SL.TZ0.15c:
Show, without calculation, that the radius of Alpha Centauri B is smaller than the radius of Alpha Centauri A.
- 16N.3.SL.TZ0.15d: Alpha Centauri A is in equilibrium at constant radius. Explain how this equilibrium is maintained.
-
16N.3.SL.TZ0.15e:
A standard Hertzsprung–Russell (HR) diagram is shown.
Using the HR diagram, draw the present position of Alpha Centauri A and its expected evolutionary path.
-
16N.3.SL.TZ0.16a:
Determine the distance from Earth to the Cepheid star in parsecs. The luminosity of the Sun is 3.8 × 1026 W. The average apparent brightness of the Cepheid star is 1.1 × 10–9 W m–2.
- 16N.3.SL.TZ0.16b: Explain why Cephids are used as standard candles.
-
17M.3.SL.TZ1.9a.ii:
Show that the mass of Theta 1 Orionis is about 40 solar masses.
-
17M.3.SL.TZ1.9c:
The Sun and Theta 1 Orionis will eventually leave the main sequence. Compare and contrast the different stages in the evolution of the two stars.
-
17M.3.SL.TZ1.10a.ii:
The present temperature of the CMB is 2.8 K. Calculate the peak wavelength of the CMB.
-
17M.3.SL.TZ2.11b:
The Hertzsprung–Russell (HR) diagram shows two main sequence stars X and Y and includes lines of constant radius. R is the radius of the Sun.

Using the mass–luminosity relation and information from the graph, determine the ratio density of star Xdensity of star Y.
-
17M.3.SL.TZ2.11c.i:
On the HR diagram in (b), draw a line to indicate the evolutionary path of star X.
-
17M.3.SL.TZ2.11c.ii:
Outline why the neutron star that is left after the supernova stage does not collapse under the action of gravitation.
-
17M.3.SL.TZ2.11c.iv:
Determine the region of the electromagnetic spectrum in which the neutron star in (c)(iii) emits most of its energy.
-
17M.3.SL.TZ2.12c.ii:
Describe how type Ia supernovae could be used to measure the distance to this galaxy.
- 17N.3.SL.TZ0.12a: State what is meant by a binary star.
-
17N.3.SL.TZ0.12b:
The peak spectral line of Sirius B has a measured wavelength of 115 nm. Show that the surface temperature of Sirius B is about 25 000 K.
-
17N.3.SL.TZ0.12c:
The mass of Sirius B is about the same mass as the Sun. The luminosity of Sirius B is 2.5 % of the luminosity of the Sun. Show, with a calculation, that Sirius B is not a main sequence star.
-
17N.3.SL.TZ0.12d.i:
Determine the radius of Sirius B in terms of the radius of the Sun.
- 17N.3.SL.TZ0.12d.ii: Identify the star type of Sirius B.
- 17N.3.SL.TZ0.12e.i: draw the approximate positions of Sirius A, labelled A and Sirius B, labelled B.
- 17N.3.SL.TZ0.12e.ii: sketch the expected evolutionary path for Sirius A.
-
18M.3.SL.TZ1.11a.i:
Suggest, using the graphs, why star X is most likely to be a main sequence star.
-
18M.3.SL.TZ1.11a.ii:
Show that the temperature of star X is approximately 10 000 K.
-
18M.3.SL.TZ1.11b.i:
Write down the luminosity of star X (LX) in terms of the luminosity of the Sun (Ls).
-
18M.3.SL.TZ1.11b.ii:
Determine the radius of star X (RX) in terms of the radius of the Sun (Rs).
-
18M.3.SL.TZ1.11b.iii:
Estimate the mass of star X (MX) in terms of the mass of the Sun (Ms).
-
18M.3.SL.TZ1.11c:
Star X is likely to evolve into a stable white dwarf star.
Outline why the radius of a white dwarf star reaches a stable value.
-
18M.3.SL.TZ2.11d.i:
draw the main sequence.
-
18M.3.SL.TZ2.11d.ii:
plot the position, using the letter P, of the main sequence star P you calculated in (b).
-
18M.3.SL.TZ2.11d.iii:
plot the position, using the letter G, of Gacrux.
-
18M.3.SL.TZ2.11e:
Discuss, with reference to its change in mass, the evolution of star P from the main sequence until its final stable phase.
-
18N.3.SL.TZ0.12a.i:
Determine the peak wavelength of the radiation emitted by Epsilon Indi.
- 18N.3.SL.TZ0.12a.ii: Using the axis, draw the variation with wavelength of the intensity of the radiation emitted by...
-
18N.3.SL.TZ0.12b:
Epsilon Indi is a main sequence star. Show that the mass of Epsilon Indi is 0.64 M⊙.
- 18N.3.SL.TZ0.12c: Describe how the chemical composition of a star may be determined.
- 18N.3.SL.TZ0.12d: Describe the stages in the evolution of Epsilon Indi from the point when it leaves the main...
-
18N.3.HL.TZ0.18a.i:
Determine the peak wavelength of the radiation emitted by Epsilon Indi.
- 18N.3.HL.TZ0.18a.ii: Using the axis, draw the variation with wavelength of the intensity of the radiation emitted by...
-
18N.3.HL.TZ0.18b:
Epsilon Indi is a main sequence star. Show that the mass of Epsilon Indi is 0.64 M⊙.
-
18N.3.HL.TZ0.18c:
The Sun will spend about nine billion years on the main sequence. Calculate how long Epsilon Indi will spend on the main sequence.
- 18N.3.HL.TZ0.18d: Describe the stages in the evolution of Epsilon Indi from the point when it leaves the main...
- 19M.3.SL.TZ2.13ai: Outline the processes that produce the change of luminosity with time of Cepheid variables.
- 19M.3.SL.TZ2.13aii: Explain how Cepheid variables are used to determine distances.
-
19M.3.SL.TZ2.13bii:
Calculate the peak surface temperature of δ-Cephei.
- 19M.3.SL.TZ2.15a: Identify, on the HR diagram, the position of the Sun. Label the position S.
- 19M.3.SL.TZ2.15b: Suggest the conditions that will cause the Sun to become a red giant.
-
19M.3.SL.TZ2.15c:
Outline why the Sun will maintain a constant radius after it becomes a white dwarf.
-
19N.3.SL.TZ0.10b(i):
The peak wavelength of radiation from Eta Cassiopeiae A is 490 nm. Show that the surface temperature of Eta Cassiopeiae A is about 6000 K.
- 19N.3.SL.TZ0.10c(i): On the HR diagram, draw the present position of Eta Cassiopeiae A.
- 19N.3.SL.TZ0.10c(ii): State the star type of Eta Cassiopeiae A.
-
19N.3.SL.TZ0.10c(iii):
Calculate the ratio mass of Eta Cassiopeiae Amass of the Sun.
-
19N.3.SL.TZ0.10c(iv):
Deduce the final evolutionary state of Eta Cassiopeiae A.
-
20N.3.SL.TZ0.17a:
Show by calculation that Eta Aquilae A is not on the main sequence.
- 20N.3.SL.TZ0.17d: Eta Aquilae A is a Cepheid variable. Explain why the brightness of Eta Aquilae A varies.
-
20N.3.HL.TZ0.22a:
Show by calculation that Eta Aquilae A is not on the main sequence.
- 20N.3.HL.TZ0.22d: Eta Aquilae A is a Cepheid variable. Explain why the brightness of Eta Aquilae A varies.
- 21N.1.SL.TZ0.30: Which is correct for a black-body radiator? A. The power it emits from a unit surface area...
D.3 – Cosmology
- 16N.3.SL.TZ0.17a: Identify two other characteristics of the CMB radiation that are predicted from the Hot Big Bang...
- 16N.3.SL.TZ0.17b: A spectral line in the hydrogen spectrum measured in the laboratory today has a wavelength of...
-
17M.3.SL.TZ1.10a.i:
State two characteristics of the cosmic microwave background (CMB) radiation.
-
17M.3.SL.TZ1.10b:
Describe how the CMB provides evidence for the Hot Big Bang model of the universe.
-
17M.3.SL.TZ1.10c.i:
Determine the distance to this galaxy using a value for the Hubble constant of H0 = 68 km s–1Mpc–1.
-
17M.3.SL.TZ1.10c.ii:
Estimate the size of the Universe relative to its present size when the light was emitted by the galaxy in (c).
-
17M.3.SL.TZ2.12a:
Describe what is meant by the Big Bang model of the universe.
-
17M.3.SL.TZ2.12b:
State two features of the cosmic microwave background (CMB) radiation which are consistent with the Big Bang model.
-
17M.3.SL.TZ2.12c.i:
Determine the distance to the galaxy in Mpc.
- 17N.3.SL.TZ0.13a: Outline one reason for the difference in wavelength.
-
17N.3.SL.TZ0.13b:
Determine the velocity of the galaxy relative to Earth.
-
18M.3.SL.TZ1.12a:
Explain how international collaboration has helped to refine this value.
-
18M.3.SL.TZ1.12b:
Estimate, in Mpc, the distance between the galaxy and the Earth.
-
18M.3.SL.TZ2.12a:
Estimate, using the data, the age of the universe. Give your answer in seconds.
-
18M.3.SL.TZ2.12b:
Identify the assumption that you made in your answer to (a).
-
18M.3.SL.TZ2.12c:
On the graph, one galaxy is labelled A. Determine the size of the universe, relative to its present size, when light from the galaxy labelled A was emitted.
-
18N.3.SL.TZ0.13a:
Outline how Hubble measured the recessional velocities of galaxies.
-
18N.3.SL.TZ0.13b:
Using the graph, determine in s, the age of the universe.
-
18N.3.HL.TZ0.19a:
Outline how Hubble measured the recessional velocities of galaxies.
-
18N.3.HL.TZ0.19b:
Use the graph to determine the age of the universe in s.
- 19M.3.SL.TZ2.14ai: A galaxy is 1.6 × 108 ly from Earth. Show that its recessional speed as measured from Earth is...
-
19M.3.SL.TZ2.14aii:
A line in the hydrogen spectrum when measured on Earth has a wavelength of 486 nm. Calculate, in nm, the wavelength of the same hydrogen line when observed in the galaxy’s emission spectrum.
- 19M.3.SL.TZ2.14b: Outline how observations of spectra from distant galaxies provide evidence that the universe is...
- 19M.3.HL.TZ1.19b: Suggest why type I a supernovae were used in the study that led to the conclusion that the...
- 19N.3.SL.TZ0.11a(i): Outline how the light spectra of distant galaxies are used to confirm hypotheses about the...
-
19N.3.SL.TZ0.11a(ii):
Light from a hydrogen source in a laboratory on Earth contains a spectral line of wavelength 122 nm. Light from the same spectral line reaching Earth from a distant galaxy has a wavelength of 392 nm. Determine the ratio of the present size of the universe to the size of the universe when the light was emitted by the galaxy.
-
19N.3.SL.TZ0.11b(i):
Estimate the age of the universe in seconds using the Hubble constant H0 = 70 km s–1 Mpc–1.
-
19N.3.SL.TZ0.11b(ii):
Outline why the estimate made in (b)(i) is unlikely to be the actual age of the universe.
- 19N.3.HL.TZ0.16a(i): Outline how the light spectra of distant galaxies are used to confirm hypotheses about the...
-
19N.3.HL.TZ0.16a(ii):
Light from a hydrogen source in a laboratory on Earth contains a spectral line of wavelength 122 nm. Light from the same spectral line reaching Earth from a distant galaxy has a wavelength of 392 nm. Determine the ratio of the present size of the universe to the size of the universe when the light was emitted by the galaxy.
-
20N.3.SL.TZ0.16a:
The light from a distant galaxy shows that z=0.11.
Calculate the ratio size of the universe when the light was emittedsize of the universe at present.
-
20N.3.SL.TZ0.16b:
Outline how Hubble’s law is related to z.
-
20N.3.HL.TZ0.21a:
The light from a distant galaxy shows that z=0.11.
Calculate the ratio size of the universe when the light was emittedsize of the universe at present.
-
20N.3.HL.TZ0.21b:
Outline how Hubble’s law is related to z.
- 21N.1.SL.TZ0.30: Which is correct for a black-body radiator? A. The power it emits from a unit surface area...
