| Date | November 2015 | Marks available | 3 | Reference code | 15N.3.HL.TZ0.12 |
| Level | Higher level | Paper | Paper 3 | Time zone | Time zone 0 |
| Command term | Calculate | Question number | 12 | Adapted from | N/A |
Question
This question is about relativistic kinematics.
A spacecraft is flying in a straight line above a base station at a speed of 0.8c.
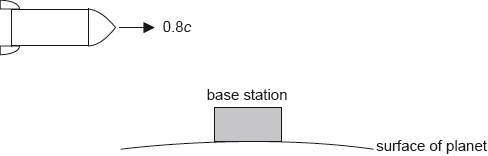
Suzanne is inside the spacecraft and Juan is on the base station.
State what is meant by an inertial frame of reference.
A light on the base station flashes regularly. According to Suzanne, the light flashes every 3 seconds. Calculate how often the light flashes according to Juan.
While moving away from the base station, Suzanne observes another spacecraft travelling towards her at a speed of 0.8c. Suzanne measures the other spacecraft to have a length of 8.00 m. Calculate the proper length of the other spacecraft.
Suzanne’s spacecraft is on a journey to a star. According to Juan, the distance from the base station to the star is 11.4 ly. Show that Suzanne measures the time taken for her to travel from the base station to the star to be about 9 years.
Suzanne then returns to the base station at the same speed. The total time since leaving the base station as measured by Suzanne is around 18 years but the total time according to Juan is around 29 years. Explain how it is possible for Suzanne and Juan to have aged by different amounts.
Markscheme
a coordinate system;
that is not accelerating / where Newton’s first law applies;
γ=[1√1−0.82=] 1.67;
Δt0=[31.67=] 1.8 (s);
velocity is relative to Suzanne / Suzanne does not measure proper length;
L0=(yL=53×8=) 13;
m;
Award first marking point even if it is implied.
Award the first marking point if the second mark is awarded.
or
accept 0.8 c with respect to the ground:
u′x=0.8c−[−0.8]c1+0.82 (=0.976c);
γ=1√1−0.9762 (=4.56);
l0=(4.56×8.0=) 36 (m);
Note: the final answer for HP3 is different to the SP3.
t=sv=11.40.8=14.25 (years);
Δt0=Δtγ=14.251.67=8.6 (years);
Allow ECF from (b).
Accept length contraction with the same result.
situation is not symmetrical;
Suzanne must undergo acceleration (when changing direction) but Juan does not;
Examiners report
This question required quite high ability to apply relativistic kinematics in standard situations and also explain the twin paradox. Well done by average and better candidates. There was a slight change made to the wording of the question 12(b)(ii) in the published paper and published markscheme in comparison with the wording used in the exam.
This question required quite high ability to apply relativistic kinematics in standard situations and also explain the twin paradox. Well done by average and better candidates. There was a slight change made to the wording of the question 12(b)(ii) in the published paper and published markscheme in comparison with the wording used in the exam.
This question required quite high ability to apply relativistic kinematics in standard situations and also explain the twin paradox. Well done by average and better candidates. There was a slight change made to the wording of the question 12(b)(ii) in the published paper and published markscheme in comparison with the wording used in the exam.
This question required quite high ability to apply relativistic kinematics in standard situations and also explain the twin paradox. Well done by average and better candidates. There was a slight change made to the wording of the question 12(b)(ii) in the published paper and published markscheme in comparison with the wording used in the exam.
This question required quite high ability to apply relativistic kinematics in standard situations and also explain the twin paradox. Well done by average and better candidates. There was a slight change made to the wording of the question 12(b)(ii) in the published paper and published markscheme in comparison with the wording used in the exam.

