| Date | May 2018 | Marks available | 2 | Reference code | 18M.2.SL.TZ1.1 |
| Level | Standard level | Paper | Paper 2 | Time zone | Time zone 1 |
| Command term | Calculate | Question number | 1 | Adapted from | N/A |
Question
An elastic climbing rope is tested by fixing one end of the rope to the top of a crane. The other end of the rope is connected to a block which is initially at position A. The block is released from rest. The mass of the rope is negligible.
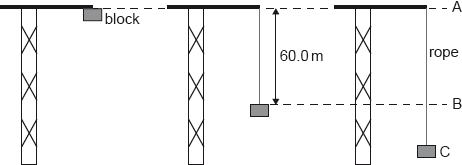
The unextended length of the rope is 60.0 m. From position A to position B, the block falls freely.
At position C the speed of the block reaches zero. The time taken for the block to fall between B and C is 0.759 s. The mass of the block is 80.0 kg.
For the rope and block, describe the energy changes that take place
At position B the rope starts to extend. Calculate the speed of the block at position B.
Determine the magnitude of the average resultant force acting on the block between B and C.
Sketch on the diagram the average resultant force acting on the block between B and C. The arrow on the diagram represents the weight of the block.
Calculate the magnitude of the average force exerted by the rope on the block between B and C.
between A and B.
between B and C.
The length reached by the rope at C is 77.4 m. Suggest how energy considerations could be used to determine the elastic constant of the rope.
Markscheme
use of conservation of energy
OR
v2 = u2 + 2as
v = «\(\sqrt {2 \times 60.0 \times 9.81} \)» = 34.3 «ms–1»
[2 marks]
use of impulse Fave × Δt = Δp
OR
use of F = ma with average acceleration
OR
F = \(\frac{{80.0 \times 34.3}}{{0.759}}\)
3620«N»
Allow ECF from (a).
[2 marks]
upwards
clearly longer than weight
For second marking point allow ECF from (b)(i) providing line is upwards.
[2 marks]
3620 + 80.0 × 9.81
4400 «N»
Allow ECF from (b)(i).
[2 marks]
(loss in) gravitational potential energy (of block) into kinetic energy (of block)
Must see names of energy (gravitational potential energy and kinetic energy) – Allow for reasonable variations of terminology (eg energy of motion for KE).
[1 mark]
(loss in) gravitational potential and kinetic energy of block into elastic potential energy of rope
See note for 1(c)(i) for naming convention.
Must see either the block or the rope (or both) mentioned in connection with the appropriate energies.
[1 mark]
k can be determined using EPE = \(\frac{1}{2}\)kx2
correct statement or equation showing
GPE at A = EPE at C
OR
(GPE + KE) at B = EPE at C
Candidate must clearly indicate the energy associated with either position A or B for MP2.
[2 marks]

