| Date | May 2017 | Marks available | 2 | Reference code | 17M.1.sl.TZ2.7 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Write down | Question number | 7 | Adapted from | N/A |
Question
A tetrahedral (four-sided) die has written on it the numbers 1, 2, 3 and 4. The die is rolled many times and the scores are noted. The table below shows the resulting frequency distribution.
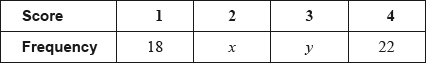
The die was rolled a total of 100 times.
The mean score is 2.71.
Write down an equation, in terms of x and y, for the total number of times the die was rolled.
Using the mean score, write down a second equation in terms of x and y.
Find the value of x and of y.
Markscheme
18+x+y+22=100 or equivalent (A1) (C1)
[1 mark]
18+2x+3y+88100=2.71 or equivalent (M1)(A1) (C2)
Note: Award (M1) for a sum including x and y, divided by 100 and equated to 2.71, (A1) for a correct equation.
[2 marks]
x+y=60 and 2x+3y=165 (M1)
Note: Award (M1) for obtaining a correct linear equation in one variable from their (a) and their (b).
This may be implied if seen in part (a) or part (b).
x=15; y=45 (A1)(ft)(A1)(ft) (C3)
Notes: Follow through from parts (a) and (b), irrespective of working seen provided the answers are positive integers.
[3 marks]

