| Date | May 2013 | Marks available | 1 | Reference code | 13M.2.sl.TZ2.7 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Show that | Question number | 7 | Adapted from | N/A |
Question
The following diagram shows a circle with centre O and radius r cm.
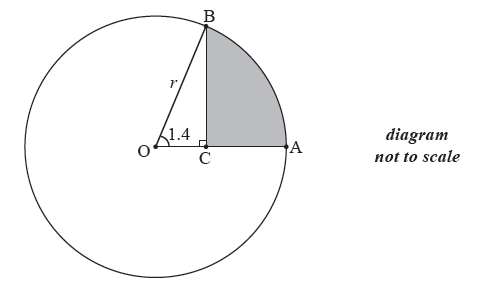
Points A and B are on the circumference of the circle and AˆOB=1.4 radians .
The point C is on [OA] such that BˆCO=π2 radians .
Show that OC=rcos1.4 .
The area of the shaded region is 25 cm2 . Find the value of r .
Markscheme
use right triangle trigonometry A1
eg cos1.4=OCr
OC=rcos1.4 AG N0
[1 mark]
correct value for BC
eg BC=rsin1.4 , √r2−(rcos1.4)2 (A1)
area of ΔOBC=12rsin1.4×rcos1.4 (=12r2sin1.4×cos1.4) A1
area of sector OAB=12r2×1.4 A1
attempt to subtract in any order (M1)
eg sector – triangle, 12r2sin1.4×cos1.4−0.7r2
correct equation A1
eg 0.7r2−12rsin1.4×rcos1.4=25
attempt to solve their equation (M1)
eg sketch, writing as quadratic, 250.616…
r=6.37 A1 N4
[7 marks]
Note: Exception to FT rule. Award A1FT for a correct FT answer from a quadratic equation involving two trigonometric functions.
Examiners report
As to be expected, candidates found this problem challenging. In part (a), many were able to use right angle trigonometry to find the length of OC.
As to be expected, candidates found this problem challenging. Those who used a systematic approach in part (b) were more successful than those whose work was scattered about the page. While a pleasing number of candidates successfully found the area of sector AOB, far fewer were able to find the area of triangle BOC. Candidates who took an analytic approach to solving the resulting equation were generally less successful than those who used their GDC. Candidates who converted the angle to degrees generally were not very successful.

