| Date | May 2012 | Marks available | 2 | Reference code | 12M.1.sl.TZ1.7 |
| Level | SL only | Paper | 1 | Time zone | TZ1 |
| Command term | Write down | Question number | 7 | Adapted from | N/A |
Question
Let f(x)=(sinx+cosx)2 .
Show that f(x) can be expressed as 1+sin2x .
The graph of f is shown below for 0≤x≤2π .
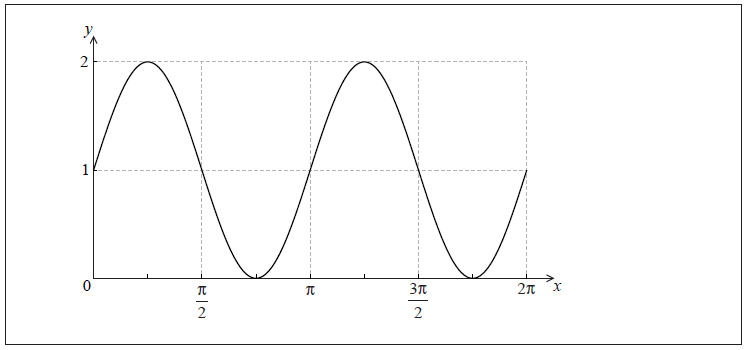
Let g(x)=1+cosx . On the same set of axes, sketch the graph of g for 0≤x≤2π .
The graph of g can be obtained from the graph of f under a horizontal stretch of scale factor p followed by a translation by the vector (k0) .
Write down the value of p and a possible value of k .
Markscheme
attempt to expand (M1)
e.g. (sinx+cosx)(sinx+cosx) ; at least 3 terms
correct expansion A1
e.g. sin2x+2sinxcosx+cos2x
f(x)=1+sin2x AG N0
[2 marks]
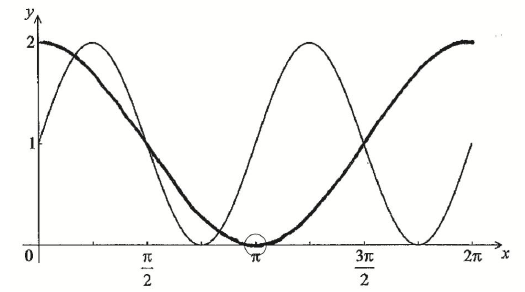 A1A1 N2
A1A1 N2
Note: Award A1 for correct sinusoidal shape with period 2π and range [0, 2], A1 for minimum in circle.
p=2 , k=−π2 A1A1 N2
[2 marks]
Examiners report
Simplifying a trigonometric expression and applying identities was generally well answered in part (a), although some candidates were certainly helped by the fact that it was a "show that" question.
More candidates had difficulty with part (b) with many assuming the first graph was 1+sin(x) and hence sketching a horizontal translation of π/2 for the graph of g; some attempts were not even sinusoidal. While some candidates found the stretch factor p correctly or from follow-through on their own graph, very few successfully found the value and direction for the translation.
Part (c) certainly served as a discriminator between the grade 6 and 7 candidates.

