| Date | May 2015 | Marks available | 3 | Reference code | 15M.2.hl.TZ2.12 |
| Level | HL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find | Question number | 12 | Adapted from | N/A |
Question
A particle moves in a straight line, its velocity v ms−1 at time t seconds is given by v=9t−3t2, 0≤t≤5.
At time t=0, the displacement s of the particle from an origin O is 3 m.
Find the displacement of the particle when t=4.
Sketch a displacement/time graph for the particle, 0≤t≤5, showing clearly where the curve meets the axes and the coordinates of the points where the displacement takes greatest and least values.
For t>5, the displacement of the particle is given by s=a+bcos2πt5 such that s is continuous for all t≥0.
Given further that s=16.5 when t=7.5, find the values of a and b.
For t>5, the displacement of the particle is given by s=a+bcos2πt5 such that s is continuous for all t≥0.
Find the times t1 and t2(0<t1<t2<8) when the particle returns to its starting point.
Markscheme
METHOD 1
s=∫(9t−3t2)dt=92t2−t3(+c) (M1)
t=0, s=3⇒c=3 (A1)
t=4⇒s=11 A1
METHOD 2
s=3+∫40(9t−3t2)dt (M1)(A1)
s=11 A1
[3 marks]
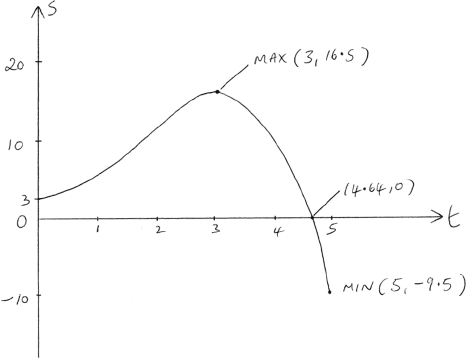
correct shape over correct domain A1
maximum at (3, 16.5) A1
t intercept at 4.64, s intercept at 3 A1
minimum at (5, −9.5) A1
[5 marks]
−9.5=a+bcos2π
16.5=a+bcos3π (M1)
Note: Only award M1 if two simultaneous equations are formed over the correct domain.
a=72 A1
b=−13 A1
[3 marks]
at t1:
3+92t2−t3=3 (M1)
t2(92−t)=0
t1=92 A1
solving 72−13cos2πt5=3 (M1)
GDC⇒t2=6.22 A1
Note: Accept graphical approaches.
[4 marks]
Total [15 marks]

