By drawing a diagram and considering the area of a suitable region under the curve, show that for $r > 0$,

$$\frac{1}{{r + 1}} < \ln \left( {\frac{{r + 1}}{r}} \right) < \frac{1}{r}.$$

Hence, given that $n$ is a positive integer greater than one, show that

$\sum\limits_{r = 1}^n {\frac{1}{r} > \ln (1 + n)}$;

Hence, given that $n$ is a positive integer greater than one, show that

$\sum\limits_{r = 1}^n {\frac{1}{r} < 1 + \ln n}$.

Hence, given that $n$ is a positive integer greater than one, show that

${U_n} > 0$;

Hence, given that $n$ is a positive integer greater than one, show that

${U_{n + 1}} < {U_n}$.

Explain why these two results prove that $\{ {U_n}\}$ is a convergent sequence.

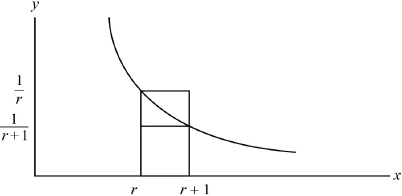
A1

Note:     Curve, both rectangles and correct $x$values required.

area of rectangles $\frac{1}{r}$ and $\frac{1}{{1 + r}}$     A1

Note:     Correct values on the $y$-axis are sufficient evidence for this mark if not otherwise indicated.

in the above diagram, the area below the curve between $x = r$ and $x = r + 1$ is between the areas of the larger and smaller rectangle

or $\frac{1}{{r + 1}} < \int\limits_r^{r + 1} {\frac{{{\text{d}}x}}{x} < \frac{1}{r}}$     (R1)

integrating, $\int_r^{r + 1} {\frac{{{\text{d}}x}}{x} = [\ln x]_r^{r + 1}\,\,\,\left( { = \ln (r + 1) - \ln (r)} \right)}$     A1

$\frac{1}{{r + 1}} < \ln \left( {\frac{{r + 1}}{r}} \right) < \frac{1}{r}$     AG

[4 marks]

summing the right-hand part of the above inequality from $r = 1$ to $r = n$,

$\sum\limits_{r = 1}^n {\frac{1}{r}} > \sum\limits_{r = 1}^n {\ln \left( {\frac{{r + 1}}{r}} \right)}$     M1

$= \ln \left( {\frac{2}{1}} \right) + \ln \left( {\frac{3}{2}} \right) + \ldots + \ln \left( {\frac{n}{{n - 1}}} \right) + \ln \left( {\frac{{n + 1}}{n}} \right)$     (A1)

EITHER

$= \ln \left( {\frac{2}{1} \times \frac{3}{2} \times \ldots \times \frac{n}{{n - 1}} \times \frac{{n + 1}}{n}} \right)$     A1

OR

$\ln 2 - \ln 1 + \ln 3 - \ln 2 + \ldots + \ln (n + 1) - \ln (n)$     A1

$= \ln (n + 1)$     AG

[3 marks]

$\sum\limits_{r = 1}^n {\frac{1}{r} = 1 + \frac{1}{2} + \frac{1}{3} + \ldots + \frac{1}{n} < 1 + \ln \left( {\frac{2}{1}} \right) + \ln \left( {\frac{3}{2}} \right) + \ldots + \ln \left( {\frac{n}{{n - 1}}} \right)}$     M1A1A1

$\left( {1 + \sum\limits_{r = 1}^{n - 1} {\frac{1}{{r + 1}} < 1 + \sum\limits_{r = 1}^{n - 1} {\ln \left( {\frac{{r + 1}}{r}} \right)} } } \right)$

Note:     M1 is for using the correct inequality from (a), A1 for both sides beginning with 1, A1 for completely correct expression.

Note:     The 1 might be added after the sums have been calculated.

$= 1 + \ln n$     AG

[3 marks]

from (b)(i) ${U_n} > \ln (1 + n) - \ln n > 0$     A1

[1 mark]

${U_{n + 1}} - {U_n} = \sum\limits_{r = 1}^{n + 1} {\frac{1}{r} - \ln (n + 1) - \sum\limits_{r = 1}^n {\frac{1}{r} + \ln n} }$     M1

$= \frac{1}{{n + 1}} - \ln \left( {\frac{{n + 1}}{n}} \right)$     A1

$< 0$ (using the result proved in (a))     A1

${U_{n + 1}} < {U_n}$     AG

[3 marks]

it follows from the two results that $\{ {U_n}\}$ cannot be divergent either in the sense of tending to $- \infty$ or oscillating therefore it must be convergent     R1

Note:     Accept the use of the result that a bounded (monotonically) decreasing sequence is convergent (allow “positive, decreasing sequence”).

[1 mark]