| Date | May 2017 | Marks available | 1 | Reference code | 17M.3dm.hl.TZ0.3 |
| Level | HL only | Paper | Paper 3 Discrete mathematics | Time zone | TZ0 |
| Command term | Explain | Question number | 3 | Adapted from | N/A |
Question
In the context of graph theory, explain briefly what is meant by a circuit;
In the context of graph theory, explain briefly what is meant by an Eulerian circuit.
The graph G has six vertices and an Eulerian circuit. Determine whether or not its complement G′ can have an Eulerian circuit.
Find an example of a graph H, with five vertices, such that H and its complement H′ both have an Eulerian trail but neither has an Eulerian circuit. Draw H and H′ as your solution.
Markscheme
a circuit is a walk that begins and ends at the same vertex and has no repeated edges A1
[1 mark]
an Eulerian circuit is a circuit that contains every edge of a graph A1
[1 mark]
if G has an Eulerian circuit all vertices are even (are of degree 2 or 4) A1
hence, G′ must have all vertices odd (of degree 1 or 3) R1
hence, G′ cannot have an Eulerian circuit R1
Note: Award A1 to candidates who begin by considering a specific G and G′ (diagram). Award R1R1 to candidates who then consider a general G and G′.
[3 marks]
for example
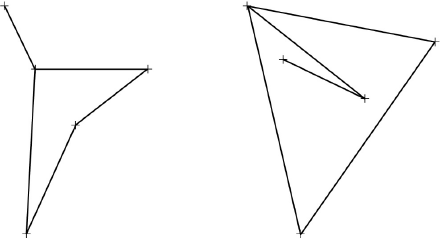
A2
A2
Notes: Each graph must have 3 vertices of order 2 and 2 odd vertices. Award A2 if one of the graphs satisfies that and the final A2 if the other graph is its complement.
[4 marks]

