(i)     Sketch the graph of $y = f(x)$ and hence justify whether or not $f$ is a bijection.

(ii)     Show that $A$ is a group under the binary operation of multiplication.

(iii)     Give a reason why $B$ is not a group under the binary operation of multiplication.

(iv)     Find an example to show that $f(a \times b) = f(a) \times f(b)$ is not satisfied for all $a,{\text{ }}b \in A$.

(i)     Sketch the graph of $y = g(x)$ and hence justify whether or not $g$ is a bijection.

(ii)     Show that $g(a + b) = g(a) \times g(b)$ for all $a,{\text{ }}b \in \mathbb{R}$.

(iii)     Given that $\{ \mathbb{R},{\text{ }} + \}$ and $\{ D,{\text{ }} \times \}$ are both groups, explain whether or not they are isomorphic.

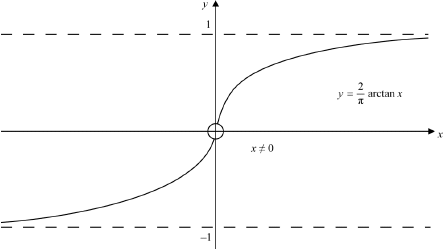(i)          A1

Notes: Award A1 for general shape, labelled asymptotes, and showing that $x \ne 0$.

graph shows that it is injective since it is increasing or by the horizontal line test     R1

graph shows that it is surjective by the horizontal line test     R1

Note: Allow any convincing reasoning.

so $f$ is a bijection     A1

(ii)     closed since non-zero real times non-zero real equals non-zero real     A1R1

we know multiplication is associative     R1

identity is 1     A1

inverse of $x$ is $\frac{1}{x}(x \ne 0)$     A1

hence it is a group     AG

(iii)     $B$ does not have an identity     A2

hence it is not a group     AG

(iv)     $f(1 \times 1) = f(1) = \frac{1}{2}$ whereas $f(1) \times f(1) = \frac{1}{2} \times \frac{1}{2} = \frac{1}{4}$ is one counterexample     A2

hence statement is not satisfied     AG

[13 marks]

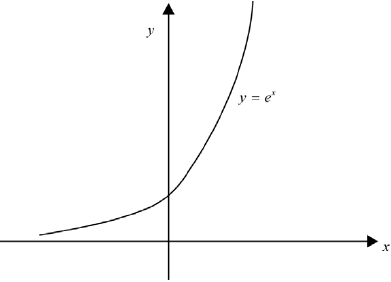
award A1 for general shape going through (0, 1) and with domain $\mathbb{R}$     A1

graph shows that it is injective since it is increasing or by the horizontal line test and graph shows that it is surjective by the horizontal line test     R1

Note: Allow any convincing reasoning.

so $g$  is a bijection     A1

(ii)     $g(a + b) = {{\text{e}}^{a + b}}$ and $g(a) \times g(b) = {{\text{e}}^a} \times {{\text{e}}^b} = {{\text{e}}^{a + b}}$     M1A1

hence $g(a + b) = g(a) \times g(b)$     AG

(iii)     since $g$ is a bijection and the homomorphism rule is obeyed     R1R1

the two groups are isomorphic     A1

[8 marks]