(i)     Sketch the set \(X \times Y\) in the Cartesian plane.

(ii)     Sketch the set \(Y \times X\) in the Cartesian plane.

(iii)     State \((X \times Y) \cap (Y \times X)\).

Consider the function \(f:X \times Y \to \mathbb{R}\) defined by \(f(x,{\text{ }}y) = x + y\) and the function \(g:X \times Y \to \mathbb{R}\) defined by \(g(x,{\text{ }}y) = xy\).

(i)     Find the range of the function f.

(ii)     Find the range of the function g.

(iii)     Show that \(f\) is an injection.

(iv)     Find \({f^{ - 1}}(\pi )\), expressing your answer in exact form.

(v)     Find all solutions to \(g(x,{\text{ }}y) = \frac{1}{2}\).

(i)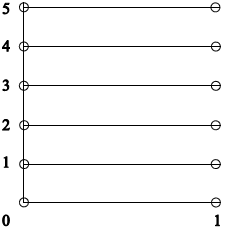     

correct horizontal lines     A1

correctly labelled axes     A1

clear indication that the endpoints are not included     A1

(ii)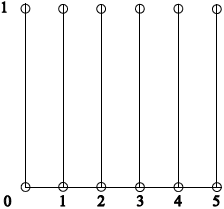     

fully correct diagram     A1

Note:     Do not penalize the inclusion of endpoints twice.

(iii)     the intersection is empty     A1

[5 marks]

(i)     range \((f) = \left] {0,{\text{ 1}}} \right[ \cup \left] {1,{\text{ 2}}} \right[ \cup \rm{L} \cup \left] {5,{\text{ 6}}} \right[\)     A1A1

Note:     A1 for six intervals and A1 for fully correct notation.

     Accept \(0 < x < 6,{\text{ }}x \ne 0{\text{, 1, 2, 3, 4, 5, 6}}\).

(ii)     range \((g) = \left[ {0,{\text{ 5}}} \right[\)     A1

(iii)     Attempt at solving

\(f({x_1},{\text{ }}{y_1}) = f({x_2},{\text{ }}{y_2})\)     M1

\(f(x,{\text{ }}y) \in \left] {y,{\text{ }}y + 1} \right[ \Rightarrow {y_1} = {y_2}\)     M1

and then \({x_1} = {x_2}\)     A1

so \(f\) is injective     AG

(iv)     \({f^{ - 1}}(\pi ) = (\pi  - 3,{\text{ }}3)\)     A1A1

(v)     solutions: (0.5, 1), (0.25, 2), \(\left( {\frac{1}{6},{\text{ 3}}} \right)\), (0.125, 4), (0.1, 5)     A2

Note:     A2 for all correct, A1 for 2 correct.

[10 marks]