| Date | November 2016 | Marks available | 2 | Reference code | 16N.3srg.hl.TZ0.1 |
| Level | HL only | Paper | Paper 3 Sets, relations and groups | Time zone | TZ0 |
| Command term | Find | Question number | 1 | Adapted from | N/A |
Question
Let {G, ∘} be the group of all permutations of 1, 2, 3, 4, 5, 6 under the operation of composition of permutations.
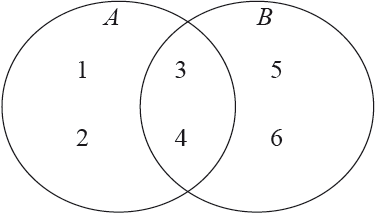
Consider the following Venn diagram, where A={1, 2, 3, 4}, B={3, 4, 5, 6}.
(i) Write the permutation α=(123456346215) as a composition of disjoint cycles.
(ii) State the order of α.
(i) Write the permutation β=(123456643512) as a composition of disjoint cycles.
(ii) State the order of β.
Write the permutation α∘β as a composition of disjoint cycles.
Write the permutation β∘α as a composition of disjoint cycles.
State the order of {G, ∘}.
Find the number of permutations in {G, ∘} which will result in A, B and A∩B remaining unchanged.
Markscheme
(i) (1 3 6 5)(2 4) A1A1
(ii) 4 A1
Note: In (b) (c) and (d) single cycles can be omitted.
[3 marks]
(i) (1 6 2 4 5)(3) A1
(ii) 5 A1
[2 marks]
(123456526134)=(1 5 3 6 4)(2) (M1)A1
[2 marks]
(123456352461)=(1 3 2 5 6)(4) (M1)A1
Note: Award A2A0 for (c) and (d) combined, if answers are the wrong way round.
[2 marks]
6!=720 A2
[2 marks]
any composition of the cycles (1 2), (3 4) and (5 6) (M1)
so 23=8 A1
[2 marks]

