| Date | November 2014 | Marks available | 2 | Reference code | 14N.3.SL.TZ0.7 |
| Level | Standard level | Paper | Paper 3 | Time zone | Time zone 0 |
| Command term | Calculate | Question number | 7 | Adapted from | N/A |
Question
This question is about radioactive decay.
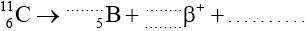
In a particular nuclear medical imaging technique, carbon-11 (116C) is used. It is radioactive and decays through β+ decay to boron (B).
The half-life of carbon-11 is 20.3 minutes.
Identify the numbers and the particle to complete the decay equation.
State the nature of the β+ particle.
Outline a method for measuring the half-life of an isotope, such as the half-life of carbon-11.
State the law of radioactive decay.
Derive the relationship between the half-life T12 and the decay constant λ , using the law of radioactive decay.
Calculate the number of nuclei of carbon-11 that will produce an activity of 4.2×1020 Bq.
Markscheme
(116C→115B+0+1β++v (or neutrino))
116C→115B+0+1β+;
v (or neutrino);
Award [1] for all the correct numbers and [1] for the neutrino.
positron / antielectron / lepton;
measure activity as a function of time;
create a graph of activity with time, and estimate half-life from the graph;
make at least three estimates of half-life from the graph and take mean;
or
measure activity as a function of time;
create a graph of ln(A) with time, find the decay constant λ from the gradient;
estimate the half-life using T12=ln2λ;
the rate of decay is proportional to the amount of (radioactive) material remaining;
the number of undecayed nuclei at time t is given by N=N0e−λt, where N0 is the number of undecayed nuclei at time t=0 and λ is the decay constant;
N02=N0e−T12;
ln(12)=−λT12 so T12=ln2λ;
λ=ln260×20.3 (=5.69×10−4s−1);
Aλ=4.2×10205.69×10−4=7.4×1023;
Examiners report
(a)(i) was well answered.
(a)(ii) was well answered.
(b)(i) was poorly answered, with many referring to measurement of the loss of mass of the sample.
(b)(ii) was very poorly answered.
Most did not use the law of radioactive decay, as required in (b)(iii).
(b)(iv) was either very well answered or very poorly answered.

