| Date | November 2014 | Marks available | 2 | Reference code | 14N.2.SL.TZ0.6 |
| Level | Standard level | Paper | Paper 2 | Time zone | Time zone 0 |
| Command term | Discuss | Question number | 6 | Adapted from | N/A |
Question
This question is in two parts. Part 1 is about energy resources. Part 2 is about electric fields.
Part 1 Energy resources
A photovoltaic panel is made up of a collection (array) of photovoltaic cells. The panel has a total area of 1.3 m2 and is mounted on the roof of a house. The maximum intensity of solar radiation at the location of the panel is 750 Wm−2. The panel produces a power output of 210 W when the solar radiation is at its maximum intensity.
The owner of the house chooses between photovoltaic panels and solar heating panels to provide 4.2 kW of power to heat water. The solar heating panels have an efficiency of 70%. The maximum intensity of solar radiation at the location remains at 750 Wm−2.
Part 2 Electric fields
An isolated metal sphere is placed in a vacuum. The sphere has a negative charge of magnitude 12 nC.

Outside the sphere, the electric field strength is equivalent to that of a point negative charge of magnitude 12 nC placed at the centre of the sphere. The radius r of the sphere is 25 mm.
An electron is initially at rest on the surface of the sphere.
The Sun is a renewable energy source whereas a fossil fuel is a non-renewable energy source. Outline the difference between renewable and non-renewable energy sources.
With reference to the energy transformations and the operation of the devices, distinguish between a photovoltaic cell and a solar heating panel.
Determine the efficiency of the photovoltaic panel.
State two reasons why the intensity of solar radiation at the location of the panel is not constant.
1.
2.
Calculate the minimum area of solar heating panel required to provide this power.
Comment on whether it is better to use a solar heating panel rather than an array of photovoltaic panels for the house. Do not consider the installation cost of the panels in your answer.
Using the diagram, draw the electric field pattern due to the charged sphere.
Show that the magnitude of the electric field strength at the surface of the sphere is about 2×105 NC−1.
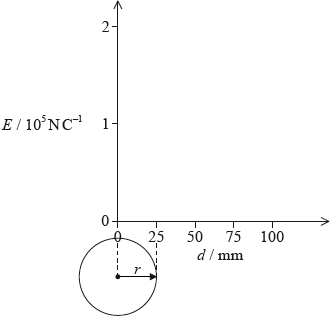
On the axes, draw a graph to show the variation of the electric field strength E with distance d from the centre of the sphere.
Calculate the initial acceleration of the electron.
Discuss the subsequent motion of the electron.
Markscheme
renewable sources:
rate of use/depletion of energy source;
is less than rate of production/regeneration of source;
Accept equivalent statement for non-renewable sources.
or
mention of rate of production / usage;
comparison of sources in terms of being used up/depleted/lasting a long time etc;
Award [1] if answer makes clear the difference but does not address the rate of production.
solar heating panel converts solar/radiation/photon/light energy into thermal/heat energy and photovoltaic cell converts solar/radiation/photon/light energy into electrical energy; } (both needed)
in solar heating hot liquid is stored/circulated and photovoltaic cell generates emf/pd; } (both needed)
(power available at roof) =1.3×750 (=975 W);
efficiency =(210975=) 0.22or22%;
depends on time of day;
depends of time of year;
depends on weather (eg cloud cover) at location;
power output of Sun varies;
Earth-Sun distance varies;
area of panel =42000.7×750;
8 m2;
calculates area of photovoltaic panels needed as about 26 m2 / makes a quantitative comparison;
solar heating takes up less area/more efficient/faster;
further energy conversion needed, from electrical to thermal, with photovoltaic panels, involving further losses / OWTTE;
Allow ECF from (d)(i) with appropriate reverse argument.
radial field with arrows and direction correct towards the sphere; (both needed)
no field inside sphere;
At least four lines of force to be shown on diagram.
use of E=kQr2;
1.73×105 NC−1; (must see answer to 2+ significant figures)
line drawn showing zero field strength inside sphere;
decreasing in inverse square-like way from a value of 2×105 NC−1or1.7×105 NC−1 at the surface, d=25 mm;
force =1.7×105×1.6×10−19; (allow use of 2×105 NC−1)
acceleration =(2.7×10−149.1×10−31=) 3.0×1016 ms−2;
radially away from sphere / away from centre of sphere;
velocity increasing but at a decreasing rate / accelerating with decreasing acceleration;
because (electric) field (strength) is decreasing;
Examiners report
It was disappointing to see some candidates sketching very imprecise lines. Most fields were radial, but often with incorrect direction.
Another “show that” question which often elicited a jumble of numbers. Line of reasoning needs to be clear. Although there were many arithmetic/POT mistakes the field strength was often given correctly.
It was extremely rare to find a zero line for the field inside of the sphere. The inverse square drop-off was often very approximate and did not always start from the surface of the sphere. The line should not touch the x-axis, but often did.
This was done correctly by a minority of candidates with many arithmetic and POT errors.
Some candidates clearly do not fully understand the difference between velocity and acceleration. It was rare to find that direction of motion was given with precision. Some candidates said that the electron would stop as the field strength approached zero.

