| Date | May 2018 | Marks available | 2 | Reference code | 18M.2.SL.TZ2.3 |
| Level | Standard level | Paper | Paper 2 | Time zone | Time zone 2 |
| Command term | Calculate | Question number | 3 | Adapted from | N/A |
Question
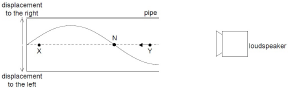
A loudspeaker emits sound towards the open end of a pipe. The other end is closed. A standing wave is formed in the pipe. The diagram represents the displacement of molecules of air in the pipe at an instant of time.
X and Y represent the equilibrium positions of two air molecules in the pipe. The arrow represents the velocity of the molecule at Y.
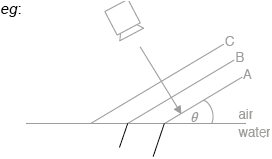
The loudspeaker in (a) now emits sound towards an air–water boundary. A, B and C are parallel wavefronts emitted by the loudspeaker. The parts of wavefronts A and B in water are not shown. Wavefront C has not yet entered the water.
Outline how the standing wave is formed.
Draw an arrow on the diagram to represent the direction of motion of the molecule at X.
Label a position N that is a node of the standing wave.
The speed of sound is 340 m s–1 and the length of the pipe is 0.30 m. Calculate, in Hz, the frequency of the sound.
The speed of sound in air is 340 m s–1 and in water it is 1500 m s–1.
The wavefronts make an angle θ with the surface of the water. Determine the maximum angle, θmax, at which the sound can enter water. Give your answer to the correct number of significant figures.
Draw lines on the diagram to complete wavefronts A and B in water for θ < θmax.
Markscheme
the incident wave «from the speaker» and the reflected wave «from the closed end»
superpose/combine/interfere
Allow superimpose/add up
Do not allow meet/interact
[1 mark]
Horizontal arrow from X to the right
MP2 is dependent on MP1
Ignore length of arrow
[1 mark]
P at a node
[1 mark]
wavelength is λ = «4×0.303 =» 0.40 «m»
f = «3400.40» 850 «Hz»
Award [2] for a bald correct answer
Allow ECF from MP1
[2 marks]
sinθc340=11500
θc = 13«°»
Award [2] for a bald correct answer
Award [2] for a bald answer of 13.1
Answer must be to 2/3 significant figures to award MP2
Allow 0.23 radians
[2 marks]
correct orientation
greater separation
Do not penalize the lengths of A and B in the water
Do not penalize a wavefront for C if it is consistent with A and B
MP1 must be awarded for MP2 to be awarded
[2 marks]

