 The changes in momentum for x and y directions are as follows (where f is final and i is inital):
The changes in momentum for x and y directions are as follows (where f is final and i is inital):
- \(\Delta p_x=mv_{x,f}-mv_{x,i}=m(-v_{x,i})-mv_{x,i}=-2mv_{x}\)
- \(\Delta p_y=mv_{y,f}-mv_{y,i}=0\)
You will notice that we have made the left direction positive for the x equation.
Since there is no change in vertical momentum against this (arbitrarily) vertical wall, we can ignore the y-component when calculating force.
 The mass of particles that will strike the wall in a given time is half of those contained within the 'blob' shaped volume shown:
The mass of particles that will strike the wall in a given time is half of those contained within the 'blob' shaped volume shown:
\(m={1\over2}\rho V={1\over 2} \rho (v_x A \Delta t )\)
We know from Newton's Second Law that force is equal to the rate of change of momentum. Per particle:
\(F={\Delta p\over \Delta t}= {-2mv_{x}\over \Delta t} \)
Substituting for m:
\(F_{wall \rightarrow particle}= {-2 {1\over 2} \rho (v_x A \Delta t )v_{x}\over \Delta t}=-\rho A {v_{x}}^2 \)
Now this force is from the wall on the particle (causing the change in momentum of the particle). Due to N3: \(F_{particle \rightarrow wall}= \rho A {v_{x}}^2\)
 Concerned about why we can ignore the y-components of speed?
Concerned about why we can ignore the y-components of speed?
For every particle that enters the region from above or below to strike the wall, there is (on average) another that will leave without striking the wall in the prescribed area.
The same applies for the z direction too as density is constant.
The ideal gas law contains pressure, not force:
\(P={F\over A}={\rho A{v_x}^2\over A}=\rho {v_x}^2\)
And considering the average speed of the particles:
\(P=\rho \bar{{v_x}^2}\)
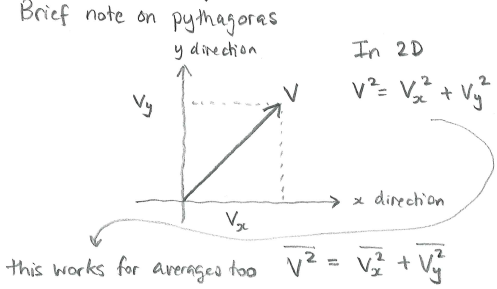
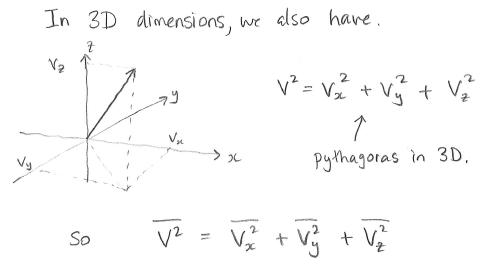
Since the particles are unaffected by gravity or any other non-contact forces, there is no preferred direction. Therefore the average speed in any direction is equal:
\(\bar {{v_x}^2}=\bar {{v_y}^2}=\bar {{v_z}^2}\)
Since \(v^2={v_x}^2+{v_y}^2+{v_z}^2\)
\(\Rightarrow v^2=3{v_x}^2\)
\(\Rightarrow {v_x}^2= {1\over 3} v^2\)
\(P={1\over 3} \rho v^2\)
Multiplying this fraction by \({2\over m} {m\over 2}\):
\(P={1\over 3} \rho {2\over m} {m\over 2} v^2 \Rightarrow P={2\over 3} {\rho \over m}E_k\)
Now, density is the ratio of mass to volume for all of the particles together:
\(\rho={nN_Am\over V}\)
\(\Rightarrow P={2\over 3}{nN_A\over V}E_k\)
In addition (and independently of the above), \(E_k={3\over2}kT\):
\(\Rightarrow P={2\over 3}{nN_A\over V}{3\over 2}kT\)
And \(R=N_Ak\):
\(PV=nRT\)
 The ideal gas equation relates pressure, temperature, volume and the amount of gas.
The ideal gas equation relates pressure, temperature, volume and the amount of gas.

 P, V and T can be plotted on a 3-D graph. In practice, however, we draw just p and V with the different temperatures represented by isotherms.
P, V and T can be plotted on a 3-D graph. In practice, however, we draw just p and V with the different temperatures represented by isotherms. If the gas is at low pressure, moderate temperature and low density, then the ideal gas law will hold.
If the gas is at low pressure, moderate temperature and low density, then the ideal gas law will hold.
 The changes in momentum for x and y directions are as follows (where f is final and i is inital):
The changes in momentum for x and y directions are as follows (where f is final and i is inital): The mass of particles that will strike the wall in a given time is half of those contained within the 'blob' shaped volume shown:
The mass of particles that will strike the wall in a given time is half of those contained within the 'blob' shaped volume shown: Concerned about why we can ignore the y-components of speed?
Concerned about why we can ignore the y-components of speed?

 Twitter
Twitter  Facebook
Facebook  LinkedIn
LinkedIn