DP Mathematics: Applications and Interpretation Questionbank

AHL 1.13—Polar and Euler form
Description
Directly related questions
-
21M.3.AHL.TZ1.1h.i:
Write down z1 and z2 in exponential form, with a constant modulus.
-
21M.3.AHL.TZ1.1h.iii:
Find, in hours, the shortest time from sunrise to sunset at point A that is predicted by this model.
-
21M.3.AHL.TZ1.1h.ii:
Hence or otherwise find an equation for L in the form L(t)=p sin(qt+r)+d, where p, q, r, d∈ℝ.
-
EXN.1.AHL.TZ0.14a:
Write down 2+5i in exponential form.
-
EXN.1.AHL.TZ0.14b:
An equilateral triangle is to be drawn on the Argand plane with one of the vertices at the point corresponding to 2+5i and all the vertices equidistant from 0.
Find the points that correspond to the other two vertices. Give your answers in Cartesian form.
-
21M.1.AHL.TZ1.9a.i:
z=2i.
-
21M.1.AHL.TZ1.9a.ii:
z=1+i.
-
21M.1.AHL.TZ1.9b:
Describe these two transformations and give the order in which they are applied.
-
21M.1.AHL.TZ1.9c:
Hence, or otherwise, find the value of z when w=2−i.
-
21M.1.AHL.TZ2.12a.ii:
Find the value of (z1z2)4 for n=2.
-
21M.1.AHL.TZ2.12a.i:
Find the value of z13.
-
21M.1.AHL.TZ2.12b:
Find the least value of n such that z1z2∈ℝ+.
-
21N.2.AHL.TZ0.5b.ii:
Hence find Re(w1+w2) in the form A cos(x-a), where A>0 and 0<a≤π2.
-
21N.2.AHL.TZ0.5a.ii:
Express z in the form z=aeib, where a, b∈ℝ, giving the exact value of a and the exact value of b.
-
21N.2.AHL.TZ0.5c.ii:
Find the phase shift of IT.
-
21N.2.AHL.TZ0.5b.i:
Find w1+w2 in the form eix(c+id).
-
21N.2.AHL.TZ0.5c.i:
Find the maximum value of IT.
-
21N.2.AHL.TZ0.5a.i:
Plot the position of z on an Argand Diagram.
-
22M.1.AHL.TZ1.10a.ii:
θ=π.
-
22M.1.AHL.TZ1.10a.i:
θ=π2.
-
22M.1.AHL.TZ1.10a.iii:
θ=3π2.
-
22M.1.AHL.TZ1.10b.i:
Find this value of θ.
-
22M.1.AHL.TZ1.10b.ii:
For this value of θ, plot the approximate position of zθ on the Argand diagram.
-
22M.1.AHL.TZ2.13a:
Find an expression for VT in the form A cos(Bt+C), where A, B and C are real constants.
-
22M.1.AHL.TZ2.13b:
Hence write down the maximum voltage in the circuit.
-
SPM.1.AHL.TZ0.15a.i:
find the values of w2, w3, and w4.
-
SPM.1.AHL.TZ0.15a.ii:
draw w, w2, w3, and w4 on the following Argand diagram.
-
SPM.1.AHL.TZ0.15b:
Let z=w2−i.
Find the value of a for which successive powers of z lie on a circle.
-
18M.1.AHL.TZ1.H_11a.i:
Express w2 and w3 in modulus-argument form.
-
18M.1.AHL.TZ1.H_11a.ii:
Sketch on an Argand diagram the points represented by w0 , w1 , w2 and w3.
-
18M.1.AHL.TZ1.H_11b:
Show that the area of the quadrilateral Q is 21√32.
-
18M.1.AHL.TZ1.H_11c:
Let z=2(cosπn+isinπn),n∈Z+. The points represented on an Argand diagram by z0,z1,z2,…,zn form the vertices of a polygon Pn.
Show that the area of the polygon Pn can be expressed in the form a(bn−1)sinπn, where a,b∈R.
-
17M.1.AHL.TZ1.H_2a.i:
By expressing z1 and z2 in modulus-argument form write down the modulus of w;
-
17M.1.AHL.TZ1.H_2a.ii:
By expressing z1 and z2 in modulus-argument form write down the argument of w.
-
17M.1.AHL.TZ1.H_2b:
Find the smallest positive integer value of n, such that wn is a real number.
-
19M.2.AHL.TZ1.H_6a:
Show the points represented by z and z−2a on the following Argand diagram.
-
17M.1.AHL.TZ2.H_11a:
Solve 2sin(x+60∘)=cos(x+30∘), 0∘⩽x⩽180∘.
-
17M.1.AHL.TZ2.H_11b:
Show that sin105∘+cos105∘=1√2.
-
17M.1.AHL.TZ2.H_11c.i:
Find the modulus and argument of z in terms of θ. Express each answer in its simplest form.
-
17M.1.AHL.TZ2.H_11c.ii:
Hence find the cube roots of z in modulus-argument form.
-
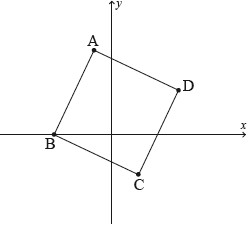
17M.1.AHL.TZ2.H_5:
In the following Argand diagram the point A represents the complex number −1+4i and the point B represents the complex number −3+0i. The shape of ABCD is a square. Determine the complex numbers represented by the points C and D.