| Date | May 2015 | Marks available | 2 | Reference code | 15M.3dm.hl.TZ0.2 |
| Level | HL only | Paper | Paper 3 Discrete mathematics | Time zone | TZ0 |
| Command term | Draw | Question number | 2 | Adapted from | N/A |
Question
The graph K2, 2 is the complete bipartite graph whose vertex set is the disjoint union of two subsets each of order two.
Draw K2, 2 as a planar graph.
Draw a spanning tree for K2, 2.
Draw the graph of the complement of K2, 2.
Show that the complement of any complete bipartite graph does not possess a spanning tree.
Markscheme
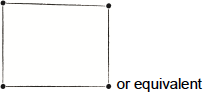 A1A1
A1A1
Note: Award A1 for a correct version of K2, 2 and A1 for a correct planar representation.
[2 marks]
 A1
A1
[1 mark]
 A1
A1
[1 mark]
the complete bipartite graph Km, n has two subsets of vertices A and B, such that each element of A is connected to every element of B A1
in the complement, no element of A is connected to any element of B. The complement is not a connected graph A1
by definition a tree is connected R1
hence the complement of any complete bipartite graph does not possess a spanning tree AG
[3 marks]
Total [7 marks]
Examiners report
Part (a) was generally well done with a large number of candidates drawing a correct planar representation for K2,2. Some candidates, however, produced a correct non-planar representation of K2,2.
Parts (b) and (c) were generally well done with many candidates drawing a correct spanning tree for K2,2 and the correct complement of K2,2.
Parts (b) and (c) were generally well done with many candidates drawing a correct spanning tree for K2,2 and the correct complement of K2,2.
Part (d) tested a candidate’s ability to produce a reasoned argument that clearly explained why the complement of Km,n does not possess a spanning tree. This was a question part in which only the best candidates provided the necessary rigour in explanation.

