| Date | May 2018 | Marks available | 2 | Reference code | 18M.3.HL.TZ1.11 |
| Level | Higher level | Paper | Paper 3 | Time zone | Time zone 1 |
| Command term | Calculate | Question number | 11 | Adapted from | N/A |
Question
The graph below represents the variation with time t of the horizontal displacement x of a mass attached to a vertical spring.
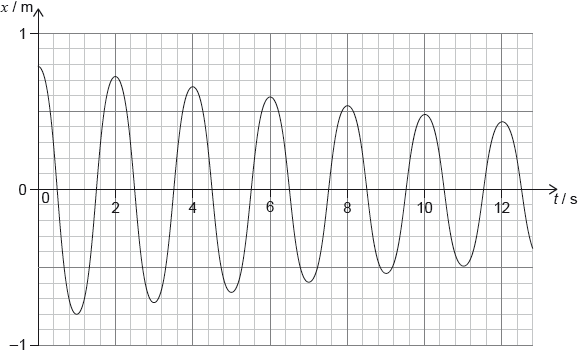
The total mass for the oscillating system is 30 kg. For this system
Describe the motion of the spring-mass system.
determine the initial energy.
calculate the Q at the start of the motion.
Markscheme
damped oscillation / OWTTE
[1 mark]
E «= \(\frac{1}{2}\) × 30 × π2 × 0.82» = 95 «J»
Allow initial amplitude between 0.77 to 0.80, giving range between: 88 to 95 J.
[1 mark]
ΔE = 95 – \(\frac{1}{2}\) × 30 × π2 × 0.722 = 18 «J»
Q = « 2π\(\frac{{95}}{{18}}\) =» 33
Accept values between 0.70 and 0.73, giving a range of ΔE between 22 and 9, giving Q between 27 and 61.
Watch for ECF from (b)(i).
[2 marks]

