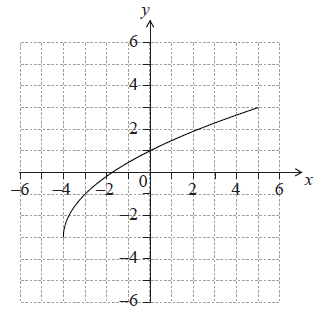
Write down the value of \(f( - 3)\).

Write down the value of  \({f^{ - 1}}(1)\).

Find the domain of \({f^{ - 1}}\).

On the grid above, sketch the graph of \({f^{ - 1}}\).

\(f( - 3) =  - 1\)     A1     N1

[1 mark]

\({f^{ - 1}}(1) = 0\)   (accept \(y = 0\))     A1     N1

[1 mark]

domain of \({f^{ - 1}}\) is range of \(f\)     (R1)

eg     \({\text{R}}f = {\text{D}}{f^{ - 1}}\)

correct answer     A1     N2

eg     \( - 3 \leqslant x \leqslant 3,{\text{ }}x \in [ - 3,{\text{ }}3]{\text{   (accept }} - 3 < x < 3,{\text{ }} - 3 \leqslant y \leqslant 3)\)

[2 marks]

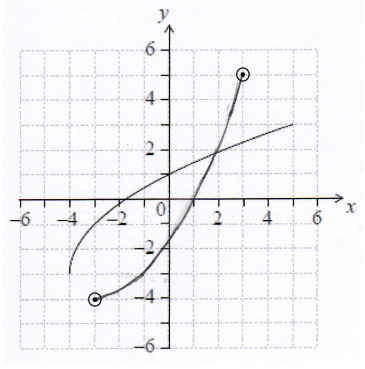     A1A1     N2

Note: Graph must be approximately correct reflection in \(y = x\).

     Only if the shape is approximately correct, award the following:

     A1 for x-intercept at \(1\), and A1 for endpoints within circles.

[2 marks]