We have all experienced circular motion. Here are some common thoughts among students...
If your speed is constant how can you be accelerating?
When you move in a circle it feels like you are being thrown out. So how do you stay in your seat?
If too many people join me on a roller coaster will we all fall to our death on the loop the loop?
Key Concepts
Rather than considering displacement, velocity and time, in this topic we will consider the angular equivalents. It is helpful to recall that circular motion is periodic, which means it repeats in cycles.
Time period (T) = time for one complete circle. Unit: s
Frequency (f) = number of complete circles per second (\(1\over T\)). Unit: Hz (s-1)
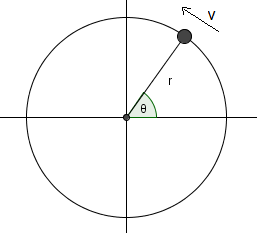
Angular displacement (θ) = angle swept out in radians. Unit: rad
Angular velocity (ω) = angle swept out per unit time. Unit: rad s-1
This could be a good time to revisit Radians.
Small angle approximation: Angle in radians = arc length/radius (\(s \over r\)). However, if the angle is small this is approximately the same as the chord length/radius.
Speed (distance / time) is related to the angular velocity.
\(v={2\pi r \over T}=\omega r\)
Note that the units are now satisfactory (it's ok to ignore radians).
The centripetal force is the resultant force acting towards the centre that makes a body travel in a circle.
\(F=m \omega^2 r={mv^2 \over r}\)
Note that, if you are asked to name the force that is acting on an object, it will not be the 'centripetal force'. This is simply the consequence of the combination of the other forces at play.
In everyday speech, we might refer to a 'centrifugal force' as flinging objects outward. In fact, this does not exist and instead is an effect of the absence of sufficient force while the system turns the corner. Relative motion does not always require a force on both objects.
How much of Circular motion have you understood?
Twitter 
Facebook 
LinkedIn