| Date | May 2018 | Marks available | 3 | Reference code | 18M.2.HL.TZ2.1 |
| Level | Higher level | Paper | Paper 2 | Time zone | 2 |
| Command term | Show that | Question number | 1 | Adapted from | N/A |
Question
The ball is now displaced through a small distance x from the bottom of the bowl and is then released from rest.
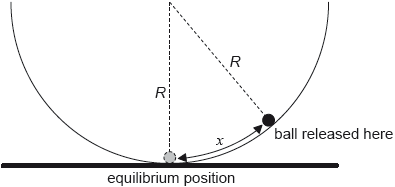
The magnitude of the force on the ball towards the equilibrium position is given by
mgxR
where R is the radius of the bowl.
A small ball of mass m is moving in a horizontal circle on the inside surface of a frictionless hemispherical bowl.

The normal reaction force N makes an angle θ to the horizontal.
State the direction of the resultant force on the ball.
On the diagram, construct an arrow of the correct length to represent the weight of the ball.
Show that the magnitude of the net force F on the ball is given by the following equation.
F=mgtanθ
The radius of the bowl is 8.0 m and θ = 22°. Determine the speed of the ball.
Outline whether this ball can move on a horizontal circular path of radius equal to the radius of the bowl.
Outline why the ball will perform simple harmonic oscillations about the equilibrium position.
Show that the period of oscillation of the ball is about 6 s.
The amplitude of oscillation is 0.12 m. On the axes, draw a graph to show the variation with time t of the velocity v of the ball during one period.
A second identical ball is placed at the bottom of the bowl and the first ball is displaced so that its height from the horizontal is equal to 8.0 m.
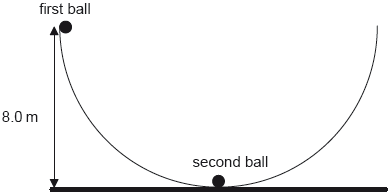
The first ball is released and eventually strikes the second ball. The two balls remain in contact. Determine, in m, the maximum height reached by the two balls.
Markscheme
towards the centre «of the circle» / horizontally to the right
Do not accept towards the centre of the bowl
[1 mark]
downward vertical arrow of any length
arrow of correct length
Judge the length of the vertical arrow by eye. The construction lines are not required. A label is not required
eg: 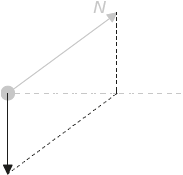
[2 marks]
ALTERNATIVE 1
F = N cos θ
mg = N sin θ
dividing/substituting to get result
ALTERNATIVE 2
right angle triangle drawn with F, N and W/mg labelled
angle correctly labelled and arrows on forces in correct directions
correct use of trigonometry leading to the required relationship
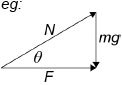
tan θ = OA=mgF
[3 marks]
mgtanθ = mv2r
r = R cos θ
v = √gRcos2θsinθ/√gRcosθtanθ/√9.81×8.0cos22tan22
v = 13.4/13 «ms –1»
Award [4] for a bald correct answer
Award [3] for an answer of 13.9/14 «ms –1». MP2 omitted
[4 marks]
there is no force to balance the weight/N is horizontal
so no / it is not possible
Must see correct justification to award MP2
[2 marks]
the «restoring» force/acceleration is proportional to displacement
Direction is not required
[1 mark]
ω = «√gR» = √9.818.0 «= 1.107 s–1»
T = «2πω = 2π1.107 =» 5.7 «s»
Allow use of or g = 9.8 or 10
Award [0] for a substitution into T = 2π√Ig
[2 marks]
sine graph
correct amplitude «0.13 m s–1»
correct period and only 1 period shown
Accept ± sine for shape of the graph. Accept 5.7 s or 6.0 s for the correct period.
Amplitude should be correct to ±12 square for MP2
eg: v /m s–1 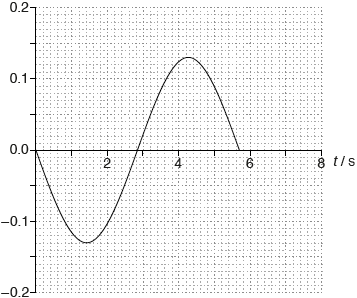
[3 marks]
speed before collision v = «√2gR =» 12.5 «ms–1»
«from conservation of momentum» common speed after collision is 12 initial speed «vc = 12.52 = 6.25 ms–1»
h = «vc22g=6.2522×9.81» 2.0 «m»
Allow 12.5 from incorrect use of kinematics equations
Award [3] for a bald correct answer
Award [0] for mg(8) = 2mgh leading to h = 4 m if done in one step.
Allow ECF from MP1
Allow ECF from MP2
[3 marks]