| Date | November 2013 | Marks available | 9 | Reference code | 13N.3ca.hl.TZ0.5 |
| Level | HL only | Paper | Paper 3 Calculus | Time zone | TZ0 |
| Command term | Justify, Sketch, State, and Write down | Question number | 5 | Adapted from | N/A |
Question
A function f is defined in the interval ]−k, k[, where k>0. The gradient function f′ exists at each point of the domain of f.
The following diagram shows the graph of y=f(x), its asymptotes and its vertical symmetry axis.
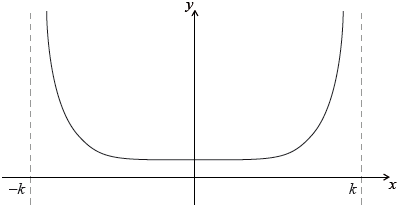
(a) Sketch the graph of y=f′(x).
Let p(x)=a+bx+cx2+dx3+… be the Maclaurin expansion of f(x).
(b) (i) Justify that a>0.
(ii) Write down a condition for the largest set of possible values for each of the parameters b, c and d.
(c) State, with a reason, an upper bound for the radius of convergence.
Markscheme
(a)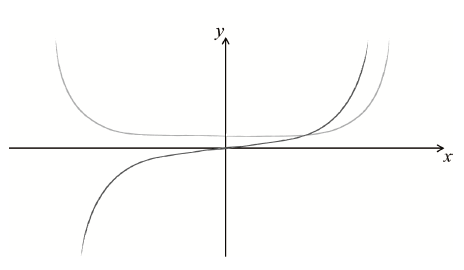
A1 for shape, A1 for passing through origin A1A1
Note: Asymptotes not required.
[2 marks]
(b) p(x)=f(0)⏟a+f′(0)⏟bx+f″
(i) because the y-intercept of f is positive R1
(ii) b = 0 A1
c \geqslant 0 A1A1
Note: A1 for > and A1 for = .
d = 0 A1
[5 marks]
(c) as the graph has vertical asymptotes x = \pm k,{\text{ }}k > 0, R1
the radius of convergence has an upper bound of k A1
Note: Accept r < k.
[2 marks]
Examiners report
Overall candidates made good attempts to parts (a) and most candidates realized that the graph contained the origin; however many candidates had difficulty rendering the correct shape of the graph of f'. Part b(i) was also well answered although some candidates where not very clear and digressed a lot. Part (ii) was less successful with most candidates scoring just part of the marks. A small number of candidates answered part (c) correctly with a valid reason.

