| Date | May 2014 | Marks available | 3 | Reference code | 14M.1.hl.TZ2.14 |
| Level | HL only | Paper | 1 | Time zone | TZ2 |
| Command term | Find, Hence, and State | Question number | 14 | Adapted from | N/A |
Question
Consider the following functions:
h(x)=arctan(x), x∈R
g(x)=1x, x∈R, x≠0
Sketch the graph of y=h(x).
Find an expression for the composite function h∘g(x) and state its domain.
Given that f(x)=h(x)+h∘g(x),
(i) find f′(x) in simplified form;
(ii) show that f(x)=π2 for x>0.
Nigel states that f is an odd function and Tom argues that f is an even function.
(i) State who is correct and justify your answer.
(ii) Hence find the value of f(x) for x<0.
Markscheme
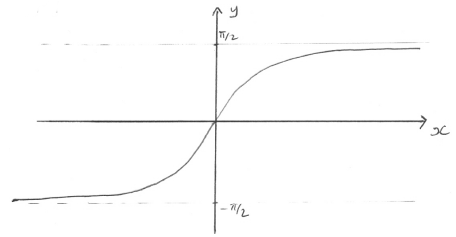 A1A1
A1A1
Note: A1 for correct shape, A1 for asymptotic behaviour at y=±π2.
[2 marks]
h∘g(x)=arctan(1x) A1
domain of h∘g is equal to the domain of g:x∈∘, x≠0 A1
[2 marks]
(i) f(x)=arctan(x)+arctan(1x)
f′(x)=11+x2+11+1x2×−1x2 M1A1
f′(x)=11+x2+−1x2x2+1x2 (A1)
=11+x2−11+x2
=0 A1
(ii) METHOD 1
f is a constant R1
when x>0
f(1)=π4+π4 M1A1
=π2 AG
METHOD 2
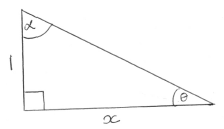
from diagram
θ=arctan1x A1
α=arctanx A1
θ+α=π2 R1
hence f(x)=π2 AG
METHOD 3
tan(f(x))=tan(arctan(x)+arctan(1x)) M1
=x+1x1−x(1x) A1
denominator = 0, so f(x)=π2 (for x>0) R1
[7 marks]
(i) Nigel is correct. A1
METHOD 1
arctan(x) is an odd function and 1x is an odd function
composition of two odd functions is an odd function and sum of two odd functions is an odd function R1
METHOD 2
f(−x)=arctan(−x)+arctan(−1x)=−arctan(x)−arctan(1x)=−f(x)
therefore f is an odd function. R1
(ii) f(x)=−π2 A1
[3 marks]

