The binary operation \(\Delta\) is defined on the set \(S =\) {1, 2, 3, 4, 5} by the following Cayley table.

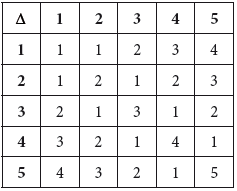
(a)     State whether S is closed under the operation Δ and justify your answer.

(b)     State whether Δ is commutative and justify your answer.

(c)     State whether there is an identity element and justify your answer.

(d)     Determine whether Δ is associative and justify your answer.

(e)     Find the solutions of the equation \(a\Delta b = 4\Delta b\), for \(a \ne 4\).

(a)     yes     A1

because the Cayley table only contains elements of S     R1

[2 marks]

(b)     yes     A1

because the Cayley table is symmetric     R1

[2 marks]

(c)     no     A1

because there is no row (and column) with 1, 2, 3, 4, 5     R1

[2 marks]

(d)     attempt to calculate \((a\Delta b)\Delta c\) and \(a\Delta (b\Delta c)\) for some \(a,{\text{ }}b,{\text{ }}c \in S\)     M1

counterexample: for example, \((1\Delta 2)\Delta 3 = 2\)

\(1\Delta (2\Delta 3) = 1\)     A1

Δ is not associative     A1

Note:     Accept a correct evaluation of \((a\Delta b)\Delta c\) and \(a\Delta (b\Delta c)\) for some \(a,{\text{ }}b,{\text{ }}c \in S\) for the M1.

[3 marks]

(e)     for example, attempt to enumerate \(4\Delta b\) for b = 1, 2, 3, 4, 5 and obtain (3, 2, 1, 4, 1)     (M1)

find \((a,{\text{ }}b) \in \left\{ {{\text{(2, 2), (2, 3)}}} \right\}\) for \(a \ne 4\) (or equivalent)     A1A1

Note: Award M1A1A0 if extra ‘solutions’ are listed.

[3 marks]

Total [12 marks]