| Date | May 2014 | Marks available | 5 | Reference code | 14M.3ca.hl.TZ0.2 |
| Level | HL only | Paper | Paper 3 Calculus | Time zone | TZ0 |
| Command term | Find and Show that | Question number | 2 | Adapted from | N/A |
Question
Consider the functions f(x)=(lnx)2, x>1 and g(x)=ln(f(x)), x>1.
(i) Find f′(x).
(ii) Find g′(x).
(iii) Hence, show that g(x) is increasing on ]1, ∞[.
Consider the differential equation
(lnx)dydx+2xy=2x−1(lnx), x>1.
(i) Find the general solution of the differential equation in the form y=h(x).
(ii) Show that the particular solution passing through the point with coordinates (e, e2) is given by y=x2−x+e(lnx)2.
(iii) Sketch the graph of your solution for x>1, clearly indicating any asymptotes and any maximum or minimum points.
Markscheme
(i) attempt at chain rule (M1)
f′(x)=2lnxx A1
(ii) attempt at chain rule (M1)
g′(x)=2xlnx A1
(iii) g′(x) is positive on ]1, ∞[ A1
so g(x) is increasing on ]1, ∞[ AG
[5 marks]
(i) rearrange in standard form:
dydx+2xlnxy=2x−1(lnx)2, x>1 (A1)
integrating factor:
e∫2xlnxdx (M1)
=eln((lnx)2)
=(lnx)2 (A1)
multiply by integrating factor (M1)
(lnx)2dydx+2lnxxy=2x−1
ddx(y(lnx)2)=2x−1 (or y(lnx)2=∫2x−1dx) M1
attempt to integrate: M1
(lnx)2y=x2−x+c
y=x2−x+c(lnx)2 A1
(ii) attempt to use the point (e, e2) to determine c: M1
eg, (lne)2e2=e2−e+c or e2=e2−e+c(lne)2 or e2=e2−e+c
c=e A1
y=x2−x+e(lnx)2 AG
(iii) 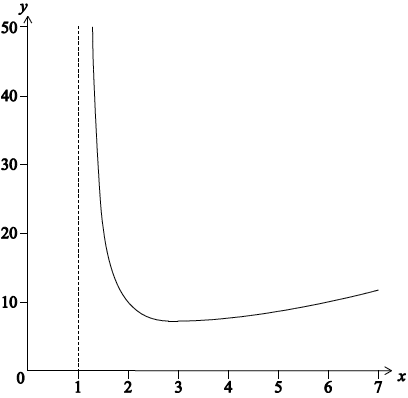
graph with correct shape A1
minimum at x=3.1 (accept answers to a minimum of 2 s.f) A1
asymptote shown at x=1 A1
Note: y-coordinate of minimum not required for A1;
Equation of asymptote not required for A1 if VA appears on the sketch.
Award A0 for asymptotes if more than one asymptote are shown
[12 marks]

