| Date | November 2016 | Marks available | 2 | Reference code | 16N.2.hl.TZ0.5 |
| Level | HL only | Paper | 2 | Time zone | TZ0 |
| Command term | State | Question number | 5 | Adapted from | N/A |
Question
Consider the function f defined by f(x)=3xarccos(x) where −1⩽.
Sketch the graph of f indicating clearly any intercepts with the axes and the coordinates of any local maximum or minimum points.
State the range of f.
Solve the inequality \left| {3x\arccos (x)} \right| > 1.
Markscheme
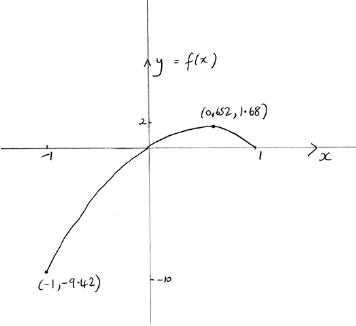
correct shape passing through the origin and correct domain A1
Note: Endpoint coordinates are not required. The domain can be indicated by - 1 and 1 marked on the axis.
(0.652,{\text{ }}1.68) A1
two correct intercepts (coordinates not required) A1
Note: A graph passing through the origin is sufficient for (0,{\text{ }}0).
[3 marks]
[-9.42,{\text{ }}1.68]{\text{ }}({\text{or }} - 3\pi ,{\text{ }}1.68]) A1A1
Note: Award A1A0 for open or semi-open intervals with correct endpoints. Award A1A0 for closed intervals with one correct endpoint.
[2 marks]
attempting to solve either \left| {3x\arccos (x)} \right| > 1 (or equivalent) or \left| {3x\arccos (x)} \right| = 1 (or equivalent) (eg. graphically) (M1)
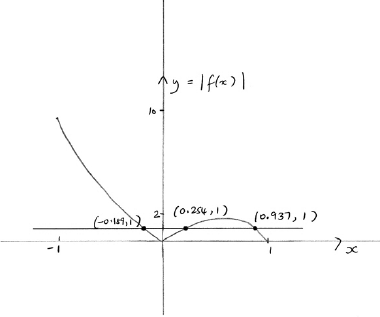
x = - 0.189,{\text{ }}0.254,{\text{ }}0.937 (A1)
- 1 \leqslant x < - 0.189{\text{ or }}0.254 < x < 0.937 A1A1
Note: Award A0 for x < - 0.189.
[4 marks]

