| Date | May 2016 | Marks available | 4 | Reference code | 16M.1.hl.TZ2.3 |
| Level | HL only | Paper | 1 | Time zone | TZ2 |
| Command term | Find | Question number | 3 | Adapted from | N/A |
Question
Show that cotα=tan(π2−α) for 0<α<π2.
Hence find ∫cotαtanα11+x2dx, 0<α<π2.
Markscheme
EITHER
use of a diagram and trig ratios
eg,
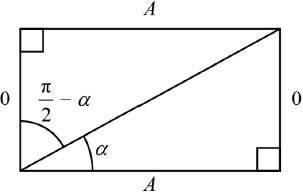
tanα=OA⇒cotα=AO
from diagram, tan(π2−α)=AO R1
OR
use of tan(π2−α)=sin(π2−α)cos(π2−α)=cosαsinα R1
THEN
cotα=tan(π2−α) AG
[1 mark]
∫cotαtanα11+x2dx=[arctanx]cotαtanα (A1)
Note: Limits (or absence of such) may be ignored at this stage.
=arctan(cotα)−arctan(tanα) (M1)
=π2−α−α (A1)
=π2−2α A1
[4 marks]
Examiners report
This was generally well done.
This was generally well done. Some weaker candidates tried to solve part (b) through use of a substitution, though the standard result arctanx was well known. A small number used arctanx+c and went on to obtain an incorrect final answer.

