| Date | November 2014 | Marks available | 2 | Reference code | 14N.3.SL.TZ0.19 |
| Level | Standard level | Paper | Paper 3 | Time zone | Time zone 0 |
| Command term | Determine | Question number | 19 | Adapted from | N/A |
Question
This question is about optic fibres.
An optic fibre consists of a thin glass fibre surrounded by a cladding material. The refractive index of the glass is 1.62.
Calculate the critical angle for this optic fibre.
The diagram shows a straight optic fibre. Sketch the passage of a ray of light through the fibre.
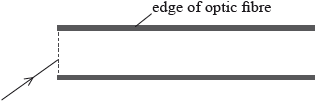
The input power to the fibre is 150 mW. The attenuation per unit length of the glass fibre is 12.0 dBkm−1. When the light has travelled a distance l its power has fallen to 3.00 mW, at which point amplification of the signal is required. Determine l.
Markscheme
(sinC=1n=11.62⇒C=) 38(.1)∘ or 0.665 rad;
rays with an angle of greater than 38∘ shown with total internal reflection;
normal drawn onto diagram for at least one point;
pairs of angles of incidence and reflection the same;
Judge by eye.
attenuation/dB=(10logI1I2=) 10log3 mW150 mWor−17.0 dB;
length=(attenuationattenuation per unit length = −17.012=) 1.42 km;
Examiners report
(a)(i) was well answered.
In (a)(ii), many candidates were not careful to obey the law of reflection.
Most failed to determine the attenuation in (b).

