| Date | November 2010 | Marks available | 3 | Reference code | 10N.3.SL.TZ0.A2 |
| Level | Standard level | Paper | Paper 3 | Time zone | Time zone 0 |
| Command term | Outline | Question number | A2 | Adapted from | N/A |
Question
This question is about diffraction and resolution.
A parallel beam of monochromatic light is incident on a narrow rectangular slit. After passing through the slit, the light is incident on a distant screen.
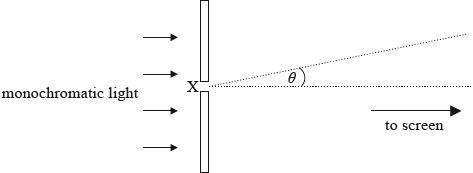
Point X is the midpoint of the slit.
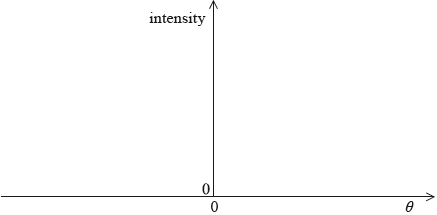
(i) On the axes below, sketch a graph to show how the intensity of the light on the screen varies with the angle θ shown in the diagram.
(ii) The wavelength of the light is 520 nm, the width of the slit is 0.04 mm and the screen is 1.2 m from the slit. Show that the width of the central maximum of intensity on the screen is about 3 cm.
Points P and Q are on the circumference of a planet as shown.
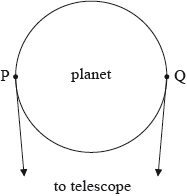
By considering the two points, outline why diffraction limits the ability of an astronomical telescope to resolve the image of the planet as a disc.
Markscheme
(i) 
general correct shape touching axis and symmetric about θ=0 (at least onesecondary maxima on each side); (judge by eye)
central maximum wider than secondary maxima;
secondary maxima at most one third intensity of central maximum;
(ii) d2=Dλb;
d=2.0×1.2×5.2×10−74.0×10−5=3.12×10−2 m;
≈3 cm
Award [2 max] for a sensible argument.
e.g. light from each point forms a diffraction pattern after being focussed by the eyepiece of the telescope;
if the diffraction patterns are not sufficiently well separated then the points will not be resolved as separate sources;
Award [1 max] for the conclusion.
e.g. if the points cannot be resolved as separate sources the planet cannot be seen as a disc;
Examiners report
Intensity distributions were often drawn well but quite a few candidates did not have their graphs in contact with the θ axis. Candidates’ working was often difficult to follow in the calculation part of this question.
Very few candidates recognised the role played by diffraction in the resolution of the planet as a disc.

