| Date | May 2017 | Marks available | 3 | Reference code | 17M.1.hl.TZ0.12 |
| Level | HL only | Paper | 1 | Time zone | TZ0 |
| Command term | Show that | Question number | 12 | Adapted from | N/A |
Question
The vertices A, B, C of an acute angled triangle have position vectors a, b, c with respect to an origin O.
The mid-point of [BC] is denoted by D. The point E lies on [AD] such that AE=2DE.
The perpendiculars from B to [AC] and C to [AB] meet at the point F.
Show that the position vector of E is
13 (a + b + c).
Explain briefly why this result shows that the three medians of a triangle are concurrent.
Show that the position vector f of F satisfies the equations
(b – f ) ∙ (c – a) = 0
(c – f ) ∙ (a – b) = 0.
Show, by expanding these equations, that
(a – f ) ∙ (c – b) = 0.
Explain briefly why this result shows that the three altitudes of a triangle are concurrent.
Markscheme

d = b + 12(c – b) = 12(b + c) (M1)A1
e = d + 13(a – d) M1
= 12(b + c) + 13(a - 12(b + c)) A1
= 13(a + b + c) AG
[??? marks]
(because of the symmetry of the result), the other two medians also pass through E. R1
[??? marks]
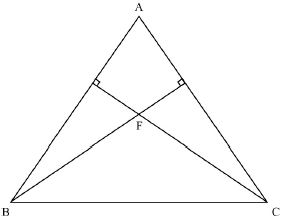
→BF = f – b and →AC = c – a A1
since FB is perpendicular to AC, (b – f) ∙ (c – a) = 0 R1AG
similarly since FC is perpendicular to BA, (c – f) ∙ (a – b) = 0 R1AG
[??? marks]
expanding these equations and adding, M1
b ∙ c – b ∙ a – f ∙ c + f ∙ a + c ∙ a – c ∙ b – f ∙ a + f ∙ b = 0 A1
– b ∙ a – f ∙ c + c ∙ a + f ∙ b = 0 A1
leading to (a – f) ∙ (c – b) = 0 AG
[??? marks]
this result shows that AF is perpendicular to BC so that the three altitudes are concurrent (at F) R1
[??? marks]

