| Date | May 2013 | Marks available | 6 | Reference code | 13M.1.hl.TZ0.3 |
| Level | HL only | Paper | 1 | Time zone | TZ0 |
| Command term | Find | Question number | 3 | Adapted from | N/A |
Question
A triangle T has sides of length 3, 4 and 5.
(i) Find the radius of the circumscribed circle of T .
(ii) Find the radius of the inscribed circle of T .
A triangle U has sides of length 4, 5 and 7.
(i) Show that the orthocentre, H, of U lies outside the triangle.
(ii) Show that the foot of the perpendicular from H to the longest side divides it in the ratio 29:20.
Markscheme
(i) T is a right angled triangle ⇒ the hypotenuse is a diameter (M1)
circumradius =2.5 A1
(ii) diagram seen with some sensible unknown(s) given (A1)

need to solve 3−r+4−r=5 (or equivalent) M1A1
r=1 A1
[6 marks]
(i) recognition that 72>42+52 M1
therefore one of the angles is obtuse R1
so the orthocentre, H, of U lies outside of the triangle AG
(ii) foot of perpendicular from H to longest side is the foot of the perpendicular from A to the longest side (R1)
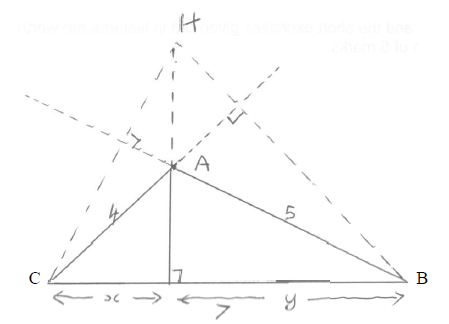
EITHER
attempt to solve 42−x2=52−(7−x)2 or 42−(7−y)2=52−y2 M1A1
obtain x=207 or y=297 A1
OR
if ˆB is the smallest angle
cosˆB=25+49−162×5×7 M1
=5870=2935
y=5×2935=297 A1
x=7−297=207 A1
THEN
ratio 29:20 (accept 20:29) AG
Note: Accept the use of Stewart’s theorem.
[6 marks]
Examiners report
A few fully correct answers were seen to this question, but many candidates were unable to make much progress after part a) (i) and a significant minority made no attempt at all. A few fully correct answers were seen to part a) (ii) and part b) (i). In both part a) (ii) and part b) (ii) a majority of candidates were unable to draw a meaningful diagram to enable them to start the question.
A few fully correct answers were seen to this question, but many candidates were unable to make much progress after part a) (i) and a significant minority made no attempt at all. A few fully correct answers were seen to part a) (ii) and part b) (i). In both part a) (ii) and part b) (ii) a majority of candidates were unable to draw a meaningful diagram to enable them to start the question.

