| Date | May 2015 | Marks available | 3 | Reference code | 15M.1.sl.TZ1.15 |
| Level | SL only | Paper | 1 | Time zone | TZ1 |
| Command term | Find | Question number | 15 | Adapted from | N/A |
Question
A cuboid has a rectangular base of width x cm and length 2x cm . The height of the cuboid is h cm . The total length of the edges of the cuboid is 72 cm.
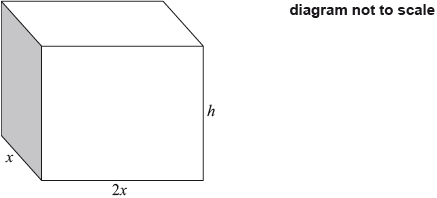
The volume, V, of the cuboid can be expressed as V=ax2−6x3.
Find the value of a.
Find the value of x that makes the volume a maximum.
Markscheme
72=12x+4h(or equivalent) (M1)
Note: Award (M1) for a correct equation obtained from the total length of the edges.
V=2x2(18−3x) (A1)
(a=) 36 (A1) (C3)
dVdx=72x−18x2 (A1)
72x−18x2=0ORdVdx=0 (M1)
Notes: Award (A1) for −18x2 seen. Award (M1) for equating derivative to zero.
(x=) 4 (A1)(ft) (C3)
Note: Follow through from part (a).
OR
Sketch of V with visible maximum (M1)
Sketch with x⩾0, V⩾0 and indication of maximum (e.g. coordinates) (A1)(ft)
(x=) 4 (A1)(ft) (C3)
Notes: Follow through from part (a).
Award (M1)(A1)(A0) for (4, 192).
Award (C3) for x=4, y=192.
Examiners report
The model in this question seemed to be too difficult for the vast majority of the candidates, and therefore was a strong discriminator between grade 6 and grade 7 candidates. An attempt to find an equation for the volume of the cube often started with V = x x 2x x h . Many struggled to translate the total length of the edges into a correct equation, and consequently were unable to substitute h. Some tried to write x in terms of h and got lost, others tried to work backwards from the expression given in the question.
As very few found a value for a, often part (b) was not attempted. When a derivative was calculated this was usually done correctly.

