| Date | May 2014 | Marks available | 2 | Reference code | 14M.2.sl.TZ2.5 |
| Level | SL only | Paper | 2 | Time zone | TZ2 |
| Command term | Find | Question number | 5 | Adapted from | N/A |
Question
A parcel is in the shape of a rectangular prism, as shown in the diagram. It has a length l cm, width w cm and height of 20 cm.
The total volume of the parcel is 3000 cm3.
Express the volume of the parcel in terms of l and w.
Show that l=150w.
The parcel is tied up using a length of string that fits exactly around the parcel, as shown in the following diagram.

Show that the length of string, S cm, required to tie up the parcel can be written as
S=40+4w+300w, 0<w⩽20.
The parcel is tied up using a length of string that fits exactly around the parcel, as shown in the following diagram.

Draw the graph of S for 0<w⩽20 and 0<S⩽500, clearly showing the local minimum point. Use a scale of 2 cm to represent 5 units on the horizontal axis w (cm), and a scale of 2 cm to represent 100 units on the vertical axis S (cm).
The parcel is tied up using a length of string that fits exactly around the parcel, as shown in the following diagram.
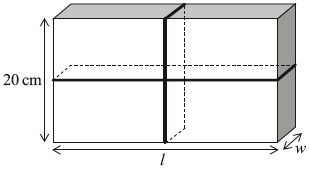
Find dSdw.
The parcel is tied up using a length of string that fits exactly around the parcel, as shown in the following diagram.
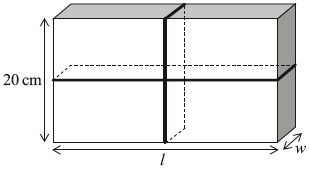
Find the value of w for which S is a minimum.
The parcel is tied up using a length of string that fits exactly around the parcel, as shown in the following diagram.
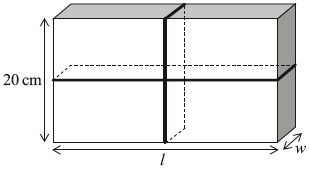
Write down the value, l, of the parcel for which the length of string is a minimum.
The parcel is tied up using a length of string that fits exactly around the parcel, as shown in the following diagram.
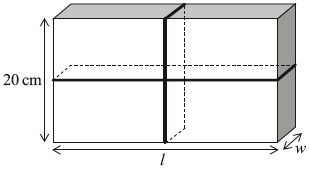
Find the minimum length of string required to tie up the parcel.
Markscheme
20lw OR V=20lw (A1)
[1 mark]
3000=20lw (M1)
Note: Award (M1) for equating their answer to part (a) to 3000.
l=300020w (M1)
Note: Award (M1) for rearranging equation to make l subject of the formula. The above equation must be seen to award (M1).
OR
150=lw (M1)
Note: Award (M1) for division by 20 on both sides. The above equation must be seen to award (M1).
l=150w (AG)
[2 marks]
S=2l+4w+2(20) (M1)
Note: Award (M1) for setting up a correct expression for S.
2(150w)+4w+2(20) (M1)
Notes: Award (M1) for correct substitution into the expression for S. The above expression must be seen to award (M1).
=40+4w+300w (AG)
[2 marks]
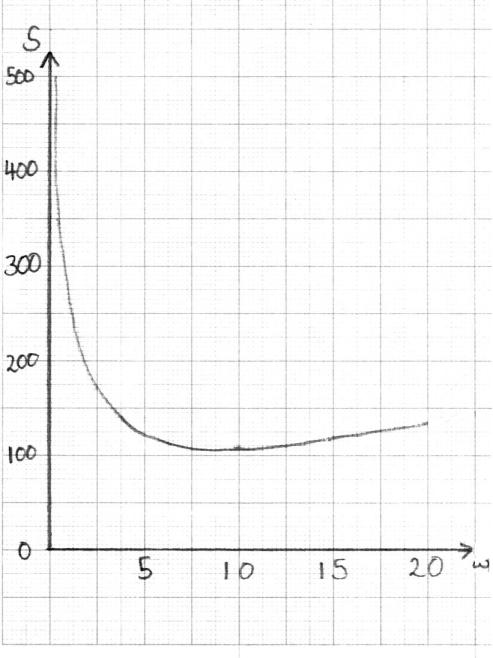 (A1)(A1)(A1)(A1)
(A1)(A1)(A1)(A1)
Note: Award (A1) for correct scales, window and labels on axes, (A1) for approximately correct shape, (A1) for minimum point in approximately correct position, (A1) for asymptotic behaviour at w=0.
Axes must be drawn with a ruler and labeled w and S.
For a smooth curve (with approximately correct shape) there should be one continuous thin line, no part of which is straight and no (one-to-many) mappings of w.
The S-axis must be an asymptote. The curve must not touch the S-axis nor must the curve approach the asymptote then deviate away later.
[4 marks]
4−300w2 (A1)(A1)(A1)
Notes: Award (A1) for 4, (A1) for −300, (A1) for 1w2 or w−2. If extra terms present, award at most (A1)(A1)(A0).
[3 marks]
4−300w2=0 OR 300w2=4 OR dSdw=0 (M1)
Note: Award (M1) for equating their derivative to zero.
w=8.66 (√75, 8.66025…) (A1)(ft)(G2)
Note: Follow through from their answer to part (e).
[2 marks]
17.3(150√75, 17.3205…) (A1)(ft)
Note: Follow through from their answer to part (f).
[1 mark]
40+4√75+300√75 (M1)
Note: Award (M1) for substitution of their answer to part (f) into the expression for S.
=110 (cm) (40+40√3, 109.282…) (A1)(ft)(G2)
Note: Do not accept 109.
Follow through from their answers to parts (f) and (g).
[2 marks]

