| Date | May 2014 | Marks available | 1 | Reference code | 14M.1.sl.TZ2.3 |
| Level | SL only | Paper | 1 | Time zone | TZ2 |
| Command term | Write down | Question number | 3 | Adapted from | N/A |
Question
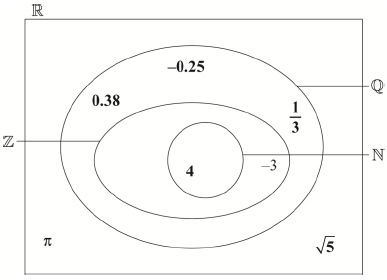
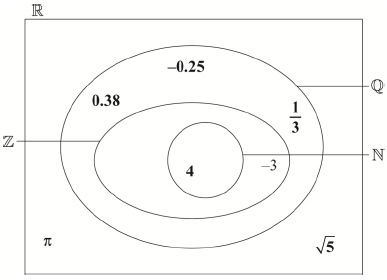
The following Venn diagram shows the relationship between the sets of numbers
N, Z, Q and R.
The number –3 belongs to the set of Z, Q and R, but not N, and is placed in the appropriate position on the Venn diagram as an example.
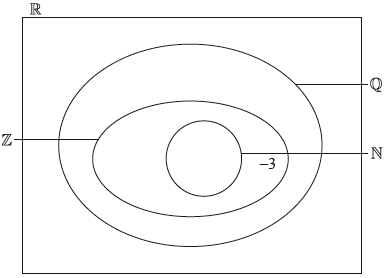
Write down the following numbers in the appropriate place in the Venn diagram.
4
13
π
0.38
√5
−0.25
Markscheme
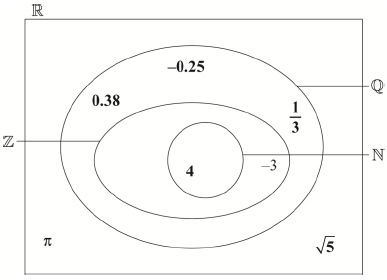 (A1)(A1)(A1)(A1)(A1)(A1) (C6)
(A1)(A1)(A1)(A1)(A1)(A1) (C6)
Note: Award (A1) for each number correctly placed.
Award (A0) for any entry in more than one region.
[1 mark]
 (A1)(A1)(A1)(A1)(A1)(A1) (C6)
(A1)(A1)(A1)(A1)(A1)(A1) (C6)
Note: Award (A1) for each number correctly placed.
Award (A0) for any entry in more than one region.
[1 mark]
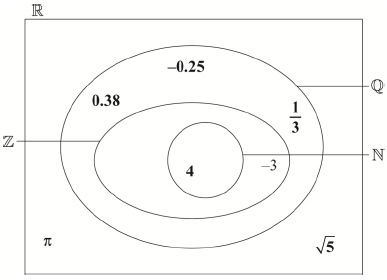 (A1)(A1)(A1)(A1)(A1)(A1) (C6)
(A1)(A1)(A1)(A1)(A1)(A1) (C6)
Note: Award (A1) for each number correctly placed.
Award (A0) for any entry in more than one region.
[1 mark]
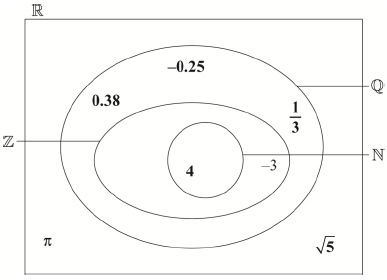 (A1)(A1)(A1)(A1)(A1)(A1) (C6)
(A1)(A1)(A1)(A1)(A1)(A1) (C6)
Note: Award (A1) for each number correctly placed.
Award (A0) for any entry in more than one region.
[1 mark]
 (A1)(A1)(A1)(A1)(A1)(A1) (C6)
(A1)(A1)(A1)(A1)(A1)(A1) (C6)
Note: Award (A1) for each number correctly placed.
Award (A0) for any entry in more than one region.
[1 mark]
 (A1)(A1)(A1)(A1)(A1)(A1) (C6)
(A1)(A1)(A1)(A1)(A1)(A1) (C6)
Note: Award (A1) for each number correctly placed.
Award (A0) for any entry in more than one region.
[1 mark]

