| Date | November 2013 | Marks available | 3 | Reference code | 13N.1.sl.TZ0.8 |
| Level | SL only | Paper | 1 | Time zone | TZ0 |
| Command term | Hence and Sketch | Question number | 8 | Adapted from | N/A |
Question
Let f(x)=3x−2 and g(x)=53x, for x≠0.
Let h(x)=5x+2, for x⩾. The graph of h has a horizontal asymptote at y = 0.
Find {f^{ - 1}}(x).
Show that \left( {g \circ {f^{ - 1}}} \right)(x) = \frac{5}{{x + 2}}.
Find the y-intercept of the graph of h.
Hence, sketch the graph of h.
For the graph of {h^{ - 1}}, write down the x-intercept;
For the graph of {h^{ - 1}}, write down the equation of the vertical asymptote.
Given that {h^{ - 1}}(a) = 3, find the value of a.
Markscheme
interchanging x and y (M1)
eg x = 3y - 2
{f^{ - 1}}(x) = \frac{{x + 2}}{3}{\text{ }}\left( {{\text{accept }}y = \frac{{x + 2}}{3},{\text{ }}\frac{{x + 2}}{3}} \right) A1 N2
[2 marks]
attempt to form composite (in any order) (M1)
eg g\left( {\frac{{x + 2}}{3}} \right),{\text{ }}\frac{{\frac{5}{{3x}} + 2}}{3}
correct substitution A1
eg \frac{5}{{3\left( {\frac{{x + 2}}{3}} \right)}}
\left( {g \circ {f^{ - 1}}} \right)(x) = \frac{5}{{x + 2}} AG N0
[2 marks]
valid approach (M1)
eg h(0),{\text{ }}\frac{5}{{0 + 2}}
y = \frac{5}{2}{\text{ }}\left( {{\text{accept (0, 2.5)}}} \right) A1 N2
[2 marks]
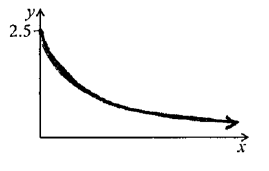 A1A2 N3
A1A2 N3
Notes: Award A1 for approximately correct shape (reciprocal, decreasing, concave up).
Only if this A1 is awarded, award A2 for all the following approximately correct features: y-intercept at (0, 2.5), asymptotic to x-axis, correct domain x \geqslant 0.
If only two of these features are correct, award A1.
[3 marks]
x = \frac{5}{2}{\text{ }}\left( {{\text{accept (2.5, 0)}}} \right) A1 N1
[1 mark]
x = 0 (must be an equation) A1 N1
[1 mark]
METHOD 1
attempt to substitute 3 into h (seen anywhere) (M1)
eg h(3),{\text{ }}\frac{5}{{3 + 2}}
correct equation (A1)
eg a = \frac{5}{{3 + 2}},{\text{ }}h(3) = a
a = 1 A1 N2
[3 marks]
METHOD 2
attempt to find inverse (may be seen in (d)) (M1)
eg x = \frac{5}{{y + 2}},{\text{ }}{h^{ - 1}} = \frac{5}{x} - 2,{\text{ }}\frac{5}{x} + 2
correct equation, \frac{5}{x} - 2 = 3 (A1)
a = 1 A1 N2
[3 marks]

