| Date | November 2015 | Marks available | 6 | Reference code | 15N.3ca.hl.TZ0.5 |
| Level | HL only | Paper | Paper 3 Calculus | Time zone | TZ0 |
| Command term | Find | Question number | 5 | Adapted from | N/A |
Question
The curves y=f(x) and y=g(x) both pass through the point (1, 0) and are defined by the differential equations dydx=x−y2 and dydx=y−x2 respectively.
Show that the tangent to the curve y=f(x) at the point (1, 0) is normal to the curve y=g(x) at the point (1, 0).
Find g(x).
Use Euler’s method with steps of 0.2 to estimate f(2) to 5 decimal places.
Explain why y=f(x) cannot cross the isocline x−y2=0, for x>1.
(i) Sketch the isoclines x−y2=−2, 0, 1.
(ii) On the same set of axes, sketch the graph of f.
Markscheme
gradient of f at (1, 0) is 1−02=1 and the gradient of g at (1, 0) is 0−12=−1 A1
so gradient of normal is 1 A1
= Gradient of the tangent of f at (1, 0) AG
[2 marks]
dydx−y=−x2
integrating factor is e∫−1dx=e−x M1
ye−x=∫−x2e−xdx A1
=x2e−x−∫2xe−xdx M1
=x2e−x+2xe−x−∫2e−xdx
=x2e−x+2xe−x+2e−x+c A1
Note: Condone missing +c at this stage.
⇒g(x)=x2+2x+2+cex
g(1)=0⇒c=−5e M1
⇒g(x)=x2+2x+2−5ex−1 A1
[6 marks]
use of yn+1=yn+hf′(xn, yn) (M1)
x0=1, y0=0
x1=1.2, y1=0.2 A1
x2=1.4, y2=0.432 (M1)(A1)
x3=1.6, y3=0.67467…
x4=1.8, y4=0.90363…
x5=2, y5=1.1003255…
answer =1.10033 A1 N3
Note: Award A0 or N1 if 1.10 given as answer.
[5 marks]
at the point (1, 0), the gradient of f is positive so the graph of f passes into the first quadrant for x>1
in the first quadrant below the curve x−y2=0 the gradient of f is positive R1
the curve x−y2=0 has positive gradient in the first quadrant R1
if f were to reach x−y2=0 it would have gradient of zero, and therefore would not cross R1
[3 marks]
(i) and (ii)
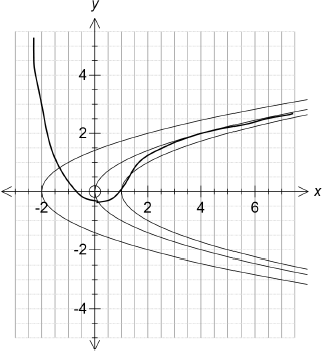 A4
A4
Note: Award A1 for 3 correct isoclines.
Award A1 for f not reaching x−y2=0.
Award A1 for turning point of f on x−y2=0.
Award A1 for negative gradient to the left of the turning point.
Note: Award A1 for correct shape and position if curve drawn without any isoclines.
[4 marks]
Total [20 marks]

