Mobile phone batteries are produced by two machines. Machine A produces 60% of the daily output and machine B produces 40%. It is found by testing that on average 2% of batteries produced by machine A are faulty and 1% of batteries produced by machine B are faulty.

(i)     Draw a tree diagram clearly showing the respective probabilities.

(ii)     A battery is selected at random. Find the probability that it is faulty.

(iii)     A battery is selected at random and found to be faulty. Find the probability that it was produced by machine A.

In a pack of seven transistors, three are found to be defective. Three transistors are selected from the pack at random without replacement. The discrete random variable X represents the number of defective transistors selected.

(i)     Find ${\text{P}}(X = 2)$.

(ii)     Copy and complete the following table:

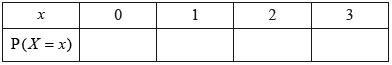
(iii)     Determine ${\text{E}}(X)$.

(i)
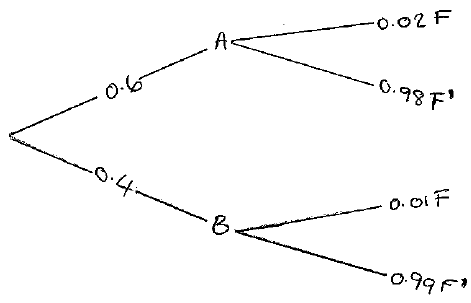     A1A1

Note:     Award A1 for a correctly labelled tree diagram and A1 for correct probabilities.

(ii)     ${\text{P}}(F) = 0.6 \times 0.02 + 0.4 \times 0.01$     (M1)

$= 0.016$     A1

(iii)     ${\text{P}}(A|F) = \frac{{{\text{P}}(A \cap F)}}{{{\text{P}}(F)}}$

$= \frac{{0.6 \times 0.02}}{{0.016}}{\text{ }}\left( { = \frac{{0.012}}{{0.016}}} \right)$     M1

$= 0.75$     A1

[6 marks]

(i)     METHOD 1

${\text{P}}(X = 2) = \frac{{^3{C_2}{ \times ^4}{C_1}}}{{^7{C_3}}}$     (M1)

$= \frac{{12}}{{35}}$     A1

METHOD 2

$\frac{3}{7} \times \frac{2}{6} \times \frac{4}{5} \times 3$     (M1)

$= \frac{{12}}{{35}}$     A1

(ii) 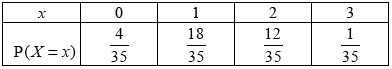         A2

Note:     Award A1 if $\frac{4}{{35}},{\text{ }}\frac{{18}}{{35}}$ or $\frac{1}{{35}}$ is obtained.

(iii)     ${\text{E}}(X) = \sum {x{\text{P}}(X = x)}$

${\text{E}}(X) = 0 \times \frac{4}{{35}} + 1 \times \frac{{18}}{{35}} + 2 \times \frac{{12}}{{35}} + 3 \times \frac{1}{{35}}$     M1

$= \frac{{45}}{{35}} = \left( {\frac{9}{7}} \right)$     A1

[6 marks]