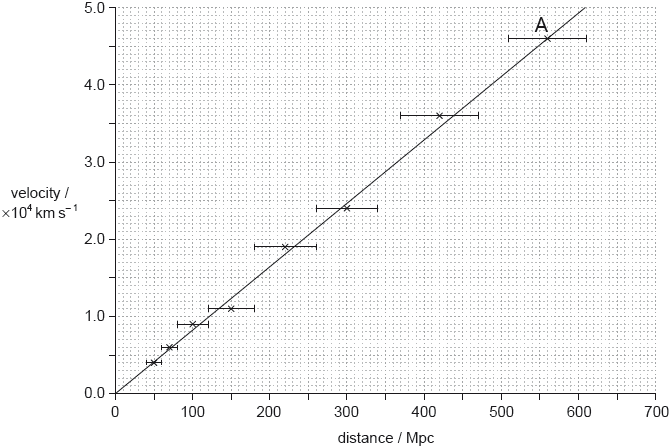
Estimate, using the data, the age of the universe. Give your answer in seconds.

Identify the assumption that you made in your answer to (a).

On the graph, one galaxy is labelled A. Determine the size of the universe, relative to its present size, when light from the galaxy labelled A was emitted.

use of gradient or any coordinate pair to find H₀ «= $\frac{v}{d}$» or $\frac{1}{{{H_0}}}$ «= $\frac{d}{v}$»

convert Mpc to m and km to m «for example $\frac{{82 \times {{10}^3}}}{{{{10}^6} \times 3.26 \times 9.46 \times {{10}^{15}}}}$»

age of universe «= $\frac{1}{{{H_0}}}$» = 3.8 × 10¹⁷ «s»

Allow final answers between

3.7 × 10¹⁷ and 3.9 × 10¹⁷ «s» or 4 × 10¹⁷ «s»

[3 marks]

non-accelerated/uniform rate of expansion

OR

H₀ constant over time

OWTTE

[1 mark]

z « = $\frac{v}{c}$» = $\frac{{4.6 \times {{10}^4} \times {{10}^3}}}{{3.00 \times {{10}^8}}}$ = 0.15

$\frac{R}{{{R_0}}}$ = «z + 1» = 1.15

$\frac{{{R_0}}}{R}$ = «$\frac{1}{{1.15}}$ =» 0.87

OR

87% of the present size

[3 marks]