| Date | May 2014 | Marks available | 14 | Reference code | 14M.2.hl.TZ0.7 |
| Level | HL only | Paper | 2 | Time zone | TZ0 |
| Command term | Find and Show that | Question number | 7 | Adapted from | N/A |
Question
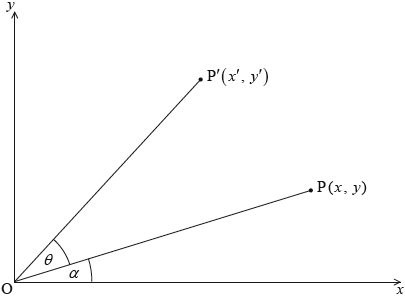
The diagram above shows the points P(x, y) and P′(x′, y′) which are equidistant from the origin O. The line (OP) is inclined at an angle α to the x-axis and PˆOP′=θ.
(a) (i) By first noting that OP=xsecα, show that x′=xcosθ−ysinθ and find a similar expression for y′.
(ii) Hence write down the 2×2 matrix which represents the anticlockwise rotation about O which takes P to P'.
(b) The ellipse E has equation 5x2+5y2−6xy=8.
(i) Show that if E is rotated clockwise about the origin through 45∘, its equation becomes x24+y2=1.
(ii) Hence determine the coordinates of the foci of E.
Markscheme
(a) (i) x′=xsecαcos(θ+α) M1
=xsecα(cosθcosα−sinθsinα) A1
=xcosθ−xtanαsinθ A1
=xcosθ−ysinθ AG
y′=xsecαsin(θ+α) M1
=xsecα(sinθcosα+cosθsinα) A1
=xsinθ+xtanαcosθ
=xsinθ+ycosθ A1
(ii) the matrix [cosθ−sinθsinθcosθ] represents the rotation A1
[7 marks]
(b) (i) the above relationship can be written in the form
[xy]=[cosθsinθ−sinθcosθ][x′y′] M1
let θ=−π4
x=x′√2−y′√2 A1
y=x′√2+y′√2
substituting in the equation of the ellipse,
5(x′√2−y′√2)2+5(x′√2+y′√2)2−6(x′√2−y′√2)(x′√2+y′√2)=8 M1
5(x′22+y′22−x′y′)+5(x′22+y′22+x′y′)−6(x′22−y′22)=8 A1
leading to x′24+y′2=1 AG
Note: Award M1A0M1A0 for using θ=π4 leading to y′24+x′2=1.
(ii) in the usual notation, a=2, b=1 (M1)
the coordinates of the foci of the rotated ellipse are (√3, 0) and (−√3, 0) A1
the coordinates of the foci of E are therefore (√3√2, √3√2) and (−√3√2, −√3√2) A1
[7 marks]

